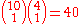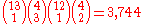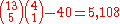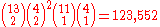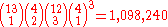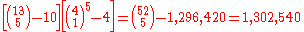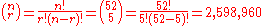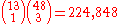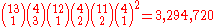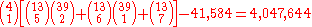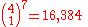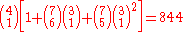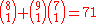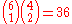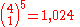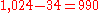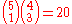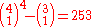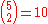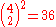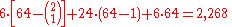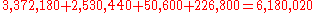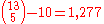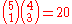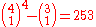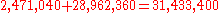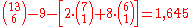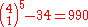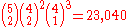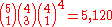Poker probability
Encyclopedia
In poker
, the probability
of each type of 5-card hand can be computed by calculating the proportion of hands of that type among all possible hands.
the (absolute) frequency
of each hand, given all combination
s of 5 cards randomly drawn from a full deck of 52 without replacement. Wild cards are not considered. The probability
of drawing a given hand is calculated by dividing the number of ways of drawing the hand by the total number of 5-card hands (the sample space,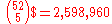
five-card hands). The odds
are defined as the ratio (1/p) - 1 : 1, where p is the probability. Note that the cumulative column contains the probability of being dealt that hand or any of the hands ranked higher than it. (The frequencies given are exact; the probabilities and odds are approximate.)
The nCr
function on most scientific calculators can be used to calculate hand frequencies; entering with and , for example, yields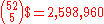
as above.
The royal flush is a case of the straight flush. It can be formed 4 ways (one for each suit), giving it a probability of 0.000154% and odds of 649,739 : 1.
When ace-low straights and ace-low straight flushes are not counted, the probabilities of each are reduced: straights and straight flushes each become 9/10 as common as they otherwise would be. The 4 missed straight flushes become flushes and the 1,020 missed straights become no pair.
Note that since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. For example, the hand 3♣ 7♣ 8♣ Q♠ A♠ is identical to 3♦ 7♦ 8♦ Q♥ A♥ because replacing all of the clubs in the first hand with diamonds and all of the spades with hearts produces the second hand. So eliminating identical hands that ignore relative suit values, there are only 134,459 distinct hands.
The number of distinct poker hands is even smaller. For example, 3♣ 7♣ 8♣ Q♠ A♠ and 3♦ 7♣ 8♦ Q♥ A♥ are not identical hands when just ignoring suit assignments because one hand has three suits, while the other hand has only two—that difference could affect the relative value of each hand when there are more cards to come. However, even though the hands are not identical from that perspective, they still form equivalent poker hands because each hand is an A-Q-8-7-3 high card hand. There are 7,462 distinct poker hands.
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
.
Any five card poker hand — The total number of five card hands that can be drawn from a deck of cards is found using a combination
selecting five cards, in any order where n refers to the number of items that can be selected and r to the sample size; the "!" is the factorial
operator:
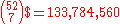. It is notable that the probability of a no-pair hand is less than the probability of a one-pair or two-pair hand.
The royal flush is not included in the cumulative probability calculation because it is a type of straight flush. The Ace-high straight flush or royal flush is slightly more frequent (4324) than the lower straight flushes (4140 each) because the remaining two cards can have any value; a King-high straight flush, for example, cannot have the Ace of its suit in the hand (as that would make it ace-high instead).
(The frequencies given are exact; the probabilities and odds are approximate.)
Since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. Eliminating identical hands that ignore relative suit values leaves 6,009,159 distinct 7-card hands.
The number of distinct 5-card poker hands that are possible from 7 cards is 4,824. Perhaps surprisingly, this is fewer than the number of 5-card poker hands from 5 cards because some 5-card hands are impossible with 7 cards (e.g. 7-high).
The following computations show how the above frequencies for 7-card poker hands were determined. To understand these derivations, the reader should be familiar with the basic properties of the binomial coefficient
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
.
, use a low hand to determine the winning hand. In most variants of lowball, the ace is counted as the lowest card and straights and flushes don't count against a low hand, so the lowest hand is the five-high hand A-2-3-4-5, also called a wheel. The probability is calculated based on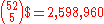
, the total number of 5-card combinations. (The frequencies given are exact; the probabilities and odds are approximate.)
As can be seen from the table, just over half the time a player gets a hand that has no pairs, three- or four-of-a-kinds. (50.7%)
If aces are not low, simply rotate the hand descriptions so that 6-high replaces 5-high for the best hand and ace-high replaces king-high as the worst hand.
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
.
The probability for any specific low hand with 5 distinct ranks (i.e. no paired cards) is the same. The frequency of a 5-high hand or any a specific low hand is calculated by making 5 independent choices for the suit for each rank, which is:
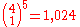
There is one way to choose the ranks for a five-high hand:
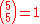
To determine the number of distinct six-high hands, once the six is chosen, the other 4 ranks are chosen from the 5 ranks A to 5, which is:
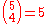
This can be generalized for any non-paired low hand. Where
is the highest rank in the hand (numbering jack–king as 11–13), the number of distinct low hands is:
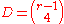
and the frequency of low hands that are
-high is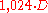 
.
Derivation for lowball hands without straights and flushes:
In the case where straights and flushes count against a low hand, the frequency of a specific hand must subtract the 4 combinations of suits that yield a flush, and the calculation for the number of distinct hands must subtract the
combinations of ranks that yield a straight. This gives the following frequency for low hands of rank 
that do not include a straight or a flush:
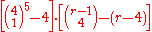
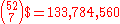, the total number of 7-card combinations.
The table does not extend to include five-card hands with at least one pair. Its "Total" represents 95.4% of the time that a player can select a 5-card low hand without any pair.
(The frequencies given are exact; the probabilities and odds are approximate.)
If aces are not low, simply rotate the hand descriptions so that 6-high replaces 5-high for the best hand and ace-high replaces king-high as the worst hand.
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
.
To make a low hand of a specific rank four ranks are chosen that are lower than the high rank. Where
is the highest rank in the hand (numbering jack–king as 11–13), the number of sets of 5 ranks that can make a low hand is:
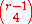
There are then three different ways to choose the remaining two cards that are not used in the low hand. Each of these cases must be considered separately:
7 distinct ranks
In this type of hand the two additional ranks are chosen from the ranks higher than
, so this type of hand can only occur when there are at least two ranks greater than 
—that is, jack-high or better hands. The suits can be assigned by making 7 independent choices for the suit for each rank, so the number of ways to make a low hand with two distinct higher ranks is:
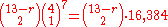
6 distinct ranks
In this type of hand there are 6 distinct ranks and one pair. The additional rank is chosen from the ranks higher than
, so this type of hand can only occur when there is at least one rank greater than 
—that is, queen-high or better hands. One of the 6 ranks is chosen for the pair and two of the four cards in that rank are chosen. The suits for the remaining 5 ranks are assigned by making 5 independent choices for each rank, so the number of ways to make a low hand with one higher ranks and a pair is:
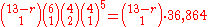
5 distinct ranks
There are two ways to choose 5 distinct ranks for seven cards. Either two pair and three unpaired ranks or three of a kind and four unpaired ranks.
Thus there are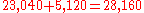
ways to make a low hand with five distinct ranks.
Derivation
Thus where
is a rank from 5 to jack (11), the total number of 
-high low hands is:
The total number of queen-high low hands is:
The total number of king-high low hands is:
Math and probability topics:
Poker
Poker is a family of card games that share betting rules and usually hand rankings. Poker games differ in how the cards are dealt, how hands may be formed, whether the high or low hand wins the pot in a showdown , limits on bet sizes, and how many rounds of betting are allowed.In most modern poker...
, the probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
of each type of 5-card hand can be computed by calculating the proportion of hands of that type among all possible hands.
Frequency of 5-card poker hands
The following enumeratesEnumeration
In mathematics and theoretical computer science, the broadest and most abstract definition of an enumeration of a set is an exact listing of all of its elements . The restrictions imposed on the type of list used depend on the branch of mathematics and the context in which one is working...
the (absolute) frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
of each hand, given all combination
Combination
In mathematics a combination is a way of selecting several things out of a larger group, where order does not matter. In smaller cases it is possible to count the number of combinations...
s of 5 cards randomly drawn from a full deck of 52 without replacement. Wild cards are not considered. The probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
of drawing a given hand is calculated by dividing the number of ways of drawing the hand by the total number of 5-card hands (the sample space,
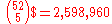five-card hands). The oddsOdds
The odds in favor of an event or a proposition are expressed as the ratio of a pair of integers, which is the ratio of the probability that an event will happen to the probability that it will not happen...
are defined as the ratio (1/p) - 1 : 1, where p is the probability. Note that the cumulative column contains the probability of being dealt that hand or any of the hands ranked higher than it. (The frequencies given are exact; the probabilities and odds are approximate.)
The nCr
Binomial coefficient
In mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
function on most scientific calculators can be used to calculate hand frequencies; entering with and , for example, yields
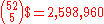as above.| Hand | Distinct Hands | Frequency | Approx. Probability | Approx. Cumulative | Approx. Odds | Mathematical expression of absolute frequency |
|---|---|---|---|---|---|---|
| Royal flush |
1 | 4 | 0.000154% | 0.000154% | 649,739 : 1 |  |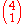
| Straight flush (excluding royal flush) |
9 | 36 | 0.00139% | 0.00154% | 72,192.33 : 1 |  |
| Four of a kind |
156 | 624 | 0.0240% | 0.0256% | 4,165 : 1 |  |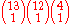
| Full house |
156 | 3,744 | 0.144% | 694.17 : 1 |  |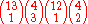
|
| Flush (excluding royal flush and straight flush) |
1,277 | 5,108 | 0.197% | 0.367% | 507.8 : 1 |  |
| Straight (excluding royal flush and straight flush) |
10 | 10,200 | 0.392% | 0.76% | 253.8 : 1 |  |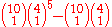
| Three of a kind |
858 | 54,912 | 2.11% | 2.87% | 46.3 : 1 |  |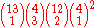
| Two pair |
858 | 123,552 | 4.75% | 7.62% | 20.03 : 1 |  |
| One pair |
2,860 | 1,098,240 | 42.3% | 49.9% | 1.36 : 1 |  |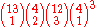
| No pair / High card |
1,277 | 1,302,540 | 50.1% | 100% | .995 : 1 |  |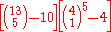
| Total | 7,462 | 2,598,960 | 100% | --- | 0 : 1 |  |
The royal flush is a case of the straight flush. It can be formed 4 ways (one for each suit), giving it a probability of 0.000154% and odds of 649,739 : 1.
When ace-low straights and ace-low straight flushes are not counted, the probabilities of each are reduced: straights and straight flushes each become 9/10 as common as they otherwise would be. The 4 missed straight flushes become flushes and the 1,020 missed straights become no pair.
Note that since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. For example, the hand 3♣ 7♣ 8♣ Q♠ A♠ is identical to 3♦ 7♦ 8♦ Q♥ A♥ because replacing all of the clubs in the first hand with diamonds and all of the spades with hearts produces the second hand. So eliminating identical hands that ignore relative suit values, there are only 134,459 distinct hands.
The number of distinct poker hands is even smaller. For example, 3♣ 7♣ 8♣ Q♠ A♠ and 3♦ 7♣ 8♦ Q♥ A♥ are not identical hands when just ignoring suit assignments because one hand has three suits, while the other hand has only two—that difference could affect the relative value of each hand when there are more cards to come. However, even though the hands are not identical from that perspective, they still form equivalent poker hands because each hand is an A-Q-8-7-3 high card hand. There are 7,462 distinct poker hands.
Derivation of frequencies of 5-card poker hands
The following computations show how the above frequencies for 5-card poker hands were determined. To understand these derivations, the reader should be familiar with the basic properties of the binomial coefficientBinomial coefficient
In mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
.
- Straight flush — Each straight flush is uniquely determined by its highest ranking card; and these ranks go from 5 (A-2-3-4-5) up to A (10-J-Q-K-A) in each of the 4 suits. Thus, the total number of straight flushes is:
- Royal straight flush — A royal straight flush is a subset of all straight flushes in which the ace is the highest card (i.e. 10-J-Q-K-A in any of the four suits). Thus, the total number of royal straight flushes is
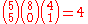
- or simply
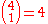. Note: this means that the total number of non-Royal straight flushes is 36.
- Four of a kind — Any one of the thirteen ranks can form the four of a kind by selecting all four of the suits in that rank. The final card can have any one of the twelve remaining ranks, and any suit. Thus, the total number of four-of-a-kinds is:
- Full house — The full house comprises a triple (three of a kind) and a pair. The triple can be any one of the thirteen ranks, and consists of three of the four suits. The pair can be any one of the remaining twelve ranks, and consists of two of the four suits. Thus, the total number of full houses is:
- Flush — The flush contains any five of the thirteen ranks, all of which belong to one of the four suits, minus the 40 straight flushes. Thus, the total number of flushes is:
- Straight — The straight consists of any one of the ten possible sequences of five consecutive cards, from 5-4-3-2-A to A-K-Q-J-10. Each of these five cards can have any one of the four suits. Finally, as with the flush, the 40 straight flushes must be excluded, giving:
- Three of a kind — Any of the thirteen ranks can form the three of a kind, which can contain any three of the four suits. The remaining two cards can have any two of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of three-of-a-kinds is:
- Two pair — The pairs can have any two of the thirteen ranks, and each pair can have two of the four suits. The final card can have any one of the eleven remaining ranks, and any suit. Thus, the total number of two-pairs is:
- Pair — The pair can have any one of the thirteen ranks, and any two of the four suits. The remaining three cards can have any three of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of pair hands is:
- No pair — A no-pair hand contains five of the thirteen ranks, discounting the ten possible straights, and each card can have any of the four suits, discounting the four possible flushes. Alternatively, a no-pair hand is any hand that does not fall into one of the above categories; that is, the complementComplement (set theory)In set theory, a complement of a set A refers to things not in , A. The relative complement of A with respect to a set B, is the set of elements in B but not in A...
of the unionUnion (set theory)In set theory, the union of a collection of sets is the set of all distinct elements in the collection. The union of a collection of sets S_1, S_2, S_3, \dots , S_n\,\! gives a set S_1 \cup S_2 \cup S_3 \cup \dots \cup S_n.- Definition :...
of all the above hands, where the universeUniverse (mathematics)In mathematics, and particularly in set theory and the foundations of mathematics, a universe is a class that contains all the entities one wishes to consider in a given situation...
is any way to choose five out of 52 cards. Thus, the total number of no-pair hands is:
Any five card poker hand — The total number of five card hands that can be drawn from a deck of cards is found using a combination
Combinatorics
Combinatorics is a branch of mathematics concerning the study of finite or countable discrete structures. Aspects of combinatorics include counting the structures of a given kind and size , deciding when certain criteria can be met, and constructing and analyzing objects meeting the criteria ,...
selecting five cards, in any order where n refers to the number of items that can be selected and r to the sample size; the "!" is the factorial
Factorial
In mathematics, the factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n...
operator:
Frequency of 7-card poker hands
In some popular variations of poker, a player uses the best five-card poker hand out of seven cards. The frequencies are calculated in a manner similar to that shown for 5-card hands, except additional complications arise due to the extra two cards in the 7-card poker hand. The total number of distinct 7-card hands is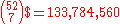. It is notable that the probability of a no-pair hand is less than the probability of a one-pair or two-pair hand.The royal flush is not included in the cumulative probability calculation because it is a type of straight flush. The Ace-high straight flush or royal flush is slightly more frequent (4324) than the lower straight flushes (4140 each) because the remaining two cards can have any value; a King-high straight flush, for example, cannot have the Ace of its suit in the hand (as that would make it ace-high instead).
| Hand | Frequency | Probability | Cumulative | Odds |
|---|---|---|---|---|
| Royal flush | 4,324 | 0.0032% | 0.0032% | 30,939 : 1 |
| Straight flush | 37,260 | 0.0279% | 0.0311% | 3,216 : 1 |
| Four of a kind | 224,848 | 0.168% | 0.199% | 594 : 1 |
| Full house | 3,473,184 | 2.60% | 2.80% | 37.5 : 1 |
| Flush | 4,047,644 | 3.03% | 5.82% | 32.1 : 1 |
| Straight | 6,180,020 | 4.62% | 10.4% | 20.6 : 1 |
| Three of a kind | 6,461,620 | 4.83% | 15.3% | 19.7 : 1 |
| Two pair | 31,433,400 | 23.5% | 38.8% | 3.26 : 1 |
| One pair | 58,627,800 | 43.8% | 82.6% | 1.28 : 1 |
| No pair | 23,294,460 | 17.4% | 100% | 4.74 : 1 |
| Total | 133,784,560 | 100% | 100% | 0 : 1 |
(The frequencies given are exact; the probabilities and odds are approximate.)
Since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. Eliminating identical hands that ignore relative suit values leaves 6,009,159 distinct 7-card hands.
The number of distinct 5-card poker hands that are possible from 7 cards is 4,824. Perhaps surprisingly, this is fewer than the number of 5-card poker hands from 5 cards because some 5-card hands are impossible with 7 cards (e.g. 7-high).
Derivation of frequencies of 7-card poker hands
See "7-Card Poker Hands" by Brian Alspach for the article on which this explanation is based.The following computations show how the above frequencies for 7-card poker hands were determined. To understand these derivations, the reader should be familiar with the basic properties of the binomial coefficient
Binomial coefficient
In mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
.
- Straight flush — Each straight flush is uniquely determined by its highest ranking card; these ranks go from 5 (A-2-3-4-5) up to A (10-J-Q-K-A) in each of the 4 suits. For any particular suit where the straight flush is ace-high, the extra 2 cards may be chosen from the remaining 47 cards. In the 9 remaining cases when the straight flush is not ace-high, the extra 2 cards may be chosen from the remaining 47 cards, minus the card in that suit directly above the high-card (which would change the rank of the hand). Thus, the total number of straight flushes is:
- Four of a kind — Any 1 of the 13 ranks can form the four of a kind, with the 3 extra cards being chosen from the remaining 48 cards. Thus, the total number of four of a kinds is:
- Full house — With 7 cards, a full house may be constructed in 1 of 3 ways:
- 1 triple, 1 pair and 2 kickers
- The triple may be 1 of 13 ranks, and by definition 3 of the 4 of that rank are chosen. The pair may be 1 of the remaining 12 ranks, and (again, by definition) 2 of the 4 of that rank are chosen. The ranks of the 2 kickers are chosen from the remaining 11 ranks, and 1 of the 4 of each rank are chosen. Thus, the total number of full houses in this form is:
- 1 triple and 2 pairs
- The triple is chosen the same way as before, the ranks of the two pairs are chosen from the remaining 12 ranks, and the 2 of the 4 of each rank are chosen as usual. Thus, the total number of full houses in this form is:
- 2 triples and 1 kicker
- The ranks of both triples are chosen from the 13, then the rank of the kicker is chosen from the remaining 11 ranks. Thus, the total number of full houses in this form is:
- Thus, the total number of full houses is:
- Flush — A flush may be formed with either 5, 6 or 7 cards in any of the 4 suits. The flush cards are chosen from the 13 in that suit, and the extra cards (if any) are chosen from the other 3 suits. The number of straight flushes must then be subtracted from the total. Thus, the total number of flushes is:
- Straight — Significantly more complications arise when working out the frequencies for a 7-card straight due to the possibility of a straight and a flush (though not necessarily a straight flush) being formed simultaneously, and the fact that pairs and triples of cards can appear. Therefore, the calculations must be broken down into several separate sections:
- 7 distinct ranks
- In this type of straight, all 7 cards are of unique ranks (ie. no pairs occur). First, ignoring suits, the total number of possible sets (combinations) that form a straight with 7 distinct ranks is found. As with straight flushes, a straight is defined by its high card. With an ace-high straight, the ranks of the 2 extra cards may be chosen from any of the remaining 8 ranks, while with the 9 other possible straights, any of the ranks but the rank directly above the high card may be chosen. Thus, the total number of sets of ranks is:
- Next, the total number of possible sets of suits, for any of the sets of ranks, is found. Given that each card is of a distinct rank, the total number of sets of suits is:
- However, the instances where a flush is formed must be subtracted from the total; there are 3 ways of achieving this: There is 1 case per suit where all 7 are of the same suit. If 6 of the 7 are in the same suit, then the remaining card is chosen from the remaining 3 suits. If 5 of the 7 in the same suit, then 2 independent choices are made for each of the extra cards. Thus, the total number of cases where a flush is formed with 7 distinct ranks is:
- Thus, the total number of sets of suits which produce a straight, but not a flush is:
- And as each set of suits occurs for each set of ranks, the total number of straights with 7 distinct ranks is:
- 6 distinct ranks
- A straight can also be formed with only 6 distinct ranks (ie. the hand contains 1 pair). In this case, one of the extra cards will have the same rank as one of the cards forming the straight, therefore only one extra rank need be chosen. Thus, the total number of sets of ranks is:
- The way to proceed now is to calculate the total number of ways to form a pair, and then calculate the total number of ways to form a straight, but not a flush (given that the pair has already been chosen). The pair can be 1 of the 6 previously chosen ranks, and 2 of the 4 of that rank form the pair. Thus, the total number of ways for form a pair is:
- The total number of sets of suits for the remaining 5 cards can be calculated in the same way as for 7 cards:
- As with 7 distinct ranks, the instances where a flush is formed must be subtracted from the total. The remaining 5 cards can be chosen in two different manners in order to form a flush: either they are all of the same suit, or 4 of them are in the same suit as either of the two paired cards. If all 5 are in the same suit, 1 of the 4 suits is chosen. If 4 of the 5 are in the same suit, 1 of the 2 suits forming the pair is chosen, and the suit of the extra card is chosen from the remaining 3 suits. Thus the total number of ways to form a flush is:
- Thus, the total number of sets of suits which produce a straight, but not a flush is:
- Thus the total number of straights with 6 distinct ranks equals the total number of sets of ranks, multiplied by the total number of ways to form the pair, multiplied by the total number of ways to form a straight:
- 5 distinct ranks with a triple
- There are two ways to form a straight with 5 distinct ranks. The first is using 3 cards of the same rank, and 4 of separate ranks. There are only 10 sets of ranks in this case, as there are no extra ranks to be chosen. The triple can be 1 of the 5 ranks, and 3 of the 4 of that rank make up the triple. Thus, the number of ways to choose the triple is:
- The total number of sets of suits for the remaining 4 cards is
and the only ways to form a flush are if all 4 cards are of the same suit as 1 of the 3 suits forming the triple. Thus, the total number of straights form a straight, but not a flush is:
- Thus the total number of straights with 5 distinct ranks and a triple is:
- 5 distinct ranks with 2 pairs
- The second way to form a straight with 5 distinct ranks is to have 2 pairs and 3 other cards of separate ranks. As before, there are 10 different sets of ranks, however, calculating the number of ways that a flush is formed is complicated, due to the fact that the two pairs can consist of either 2, 3 or 4 suits. Firstly, the ranks for the two pairs are chosen from the 5 available. Thus, the number of ways to choose the ranks for the two pairs is:
- Then the cards are chosen for each of the pairs. Thus, the number of ways to choose the suits for the pairs is:
- 6 of these ways, the pairs consist of 2 suits, 24 of these ways the pairs consist of 3 suits, and the remaining 6 of these ways they consist of 4 suits. Note that the total number of sets of suits for the remaining 3 cards is
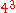. When the pairs consist of 2 suits, a flush will be formed when the remaining 3 cards are all in either of those two suits. There are 2 ways of this happening which must be subtracted from the total. When there are 3 suits, a flush will be formed when the remaining 3 cards are all in the suit of the 2 cards of matching suit in the pairs. There is 1 way of this happening. When there are 4 suits there are no ways of making a flush. Thus, the total number of sets of suits that do not form a flush is:
- Thus, the total number of straights with 5 distinct ranks and 2 pairs is:
- Thus, the total number of straights is:
- Three of a kind — A three of a kind must consist of 5 of the 13 ranks, but the 10 combinations that form straights must be subtracted, giving the total number of sets of ranks as:
- The rank of the triple is chosen from the 5 available, and 3 of the 4 of that rank are chosen. Thus, the total number of ways of choosing the triple is:
- There are
ways to choose the suits of the remaining 4 cards, minus the ways in which all 4 match one of the 3 suits in the triple (making a flush):
- Thus, the total number of three of a kinds is:
- Two pair — A two pair can be formed in 2 ways:
- 3 pairs with 1 kicker
- The 4 ranks are chosen, then 3 of the 4 are chosen for the 3 pairs, and 2 of the 4 of each rank are chosen for each pair. The kicker is then chosen from the 4 cards in the remaining rank. Thus, the total number of 3 pairs with 1 kicker is:
- 2 pairs with 3 kickers
- A two pair hand must consist of 5 of the 13 ranks, but the 10 combinations that form straights must be subtracted. 2 of the ranks are chosen for the pairs and as with the calculations for straights with 5 ranks and two pairs, there are 2,268 sets of suits that do not form flushes. Thus, the total number of 2 pairs with 3 kickers is:
- Thus, the total number of two pairs is:
- Pair — A pair hand must consist of 6 of the 13 ranks, but the combinations that form straights must be subtracted. There are 9 ways to form a 6-card straight (6- to ace-high). With 5-card straights, when the straight is either 5- or ace-high, the remaining card may be selected from any of the 8 other ranks, minus the rank at the open end of the straight (6 and 9 respectively). In any of the other 8 situations, the remaining card may be selected from any of the other 8 ranks, minus the two ranks at either end of the straight. Thus, the total number of sets of ranks that do not form straights is:
- There are
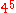ways of choosing the ranks of the kickers, and as with the calculations for straights with 6 distinct suits, there are 34 sets of suits that form flushes, therefore the total number of sets of suits that do not form flushes is:
- There are 6 different ranks to choose for the pair and the pair can be formed from 2 of the 4 cards in that rank, therefore the number of ways to choose the pair is:
- Thus, the total number of pair hands is:
- No pair — The 7 ranks are chosen, but the combinations that form straights must be subtracted. There are 8 ways to form a 7-card straight (7- to ace-high). With 6-card straights, as with 5-card straights in the pair hand calculations, any of the remaining ranks minus 1 may be chosen for the highest and lowest straight (6 ranks), while in the other cases, any remaining rank minus 2 may be chosen (5 ranks). With 5-card straights, the calculations are the same as with pairs, but 2 cards must be chosen rather than 1. Thus, the total number of sets of ranks that do not form straights is:
- There are
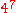ways of choosing the suits of the cards, and as with the calculations for straights with 7 distinct suits, there are 844 sets of suits that form flushes, therefore the total number of sets of suits that do not form flushes is:
- Thus, the total number of no pair hands is:
Frequency of 5-card lowball poker hands
Some variants of poker, called lowballLowball (poker)
Some forms of poker, often called lowball, sometimes called low poker, reward poor poker hands . There are four common variations on this idea, differing in whether aces are treated as high cards or low cards, and whether or not straights and flushes are used. The methods are:* Ace-to-five low:...
, use a low hand to determine the winning hand. In most variants of lowball, the ace is counted as the lowest card and straights and flushes don't count against a low hand, so the lowest hand is the five-high hand A-2-3-4-5, also called a wheel. The probability is calculated based on
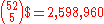, the total number of 5-card combinations. (The frequencies given are exact; the probabilities and odds are approximate.)| Hand | Distinct hands | Frequency | Probability | Cumulative | Odds |
|---|---|---|---|---|---|
| 5-high | 1 | 1,024 | 0.0394% | 0.0394% | 2,537.05 : 1 |
| 6-high | 5 | 5,120 | 0.197% | 0.236% | 506.61 : 1 |
| 7-high | 15 | 15,360 | 0.591% | 0.827% | 168.20 : 1 |
| 8-high | 35 | 35,840 | 1.38% | 2.21% | 71.52 : 1 |
| 9-high | 70 | 71,680 | 2.76% | 4.96% | 35.26 : 1 |
| 10-high | 126 | 129,024 | 4.96% | 9.93% | 19.14 : 1 |
| Jack-high | 210 | 215,040 | 8.27% | 18.2% | 11.09 : 1 |
| Queen-high | 330 | 337,920 | 13.0% | 31.2% | 6.69 : 1 |
| King-high | 495 | 506,880 | 19.5% | 50.7% | 4.13 : 1 |
| Total | 1,287 | 1,317,888 | 50.7% | 50.7% | 0.97 : 1 |
As can be seen from the table, just over half the time a player gets a hand that has no pairs, three- or four-of-a-kinds. (50.7%)
If aces are not low, simply rotate the hand descriptions so that 6-high replaces 5-high for the best hand and ace-high replaces king-high as the worst hand.
Derivation of frequencies for 5-card lowball hands
The following computations show how the above frequencies for 5-card lowball poker hands were determined. To understand these derivations, the reader should be familiar with the basic properties of the binomial coefficientBinomial coefficient
In mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
.
The probability for any specific low hand with 5 distinct ranks (i.e. no paired cards) is the same. The frequency of a 5-high hand or any a specific low hand is calculated by making 5 independent choices for the suit for each rank, which is:
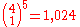
There is one way to choose the ranks for a five-high hand:
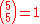
To determine the number of distinct six-high hands, once the six is chosen, the other 4 ranks are chosen from the 5 ranks A to 5, which is:
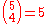
This can be generalized for any non-paired low hand. Where
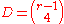
is the highest rank in the hand (numbering jack–king as 11–13), the number of distinct low hands is:and the frequency of low hands that are
-high is 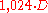.Derivation for lowball hands without straights and flushes:
In the case where straights and flushes count against a low hand, the frequency of a specific hand must subtract the 4 combinations of suits that yield a flush, and the calculation for the number of distinct hands must subtract the
combinations of ranks that yield a straight. This gives the following frequency for low hands of rank that do not include a straight or a flush: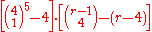Frequency of 7-card lowball poker hands
In some variants of poker a player uses the best five-card low hand selected from seven cards. In most variants of lowball, the ace is counted as the lowest card and straights and flushes don't count against a low hand, so the lowest hand is the five-high hand A-2-3-4-5, also called a wheel. The probability is calculated based on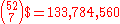, the total number of 7-card combinations.The table does not extend to include five-card hands with at least one pair. Its "Total" represents 95.4% of the time that a player can select a 5-card low hand without any pair.
| Hand | Frequency | Probability | Cumulative | Odds |
|---|---|---|---|---|
| 5-high | 781,824 | 0.584% | 0.584% | 170.12 : 1 |
| 6-high | 3,151,360 | 2.36% | 2.94% | 41.45 : 1 |
| 7-high | 7,426,560 | 5.55% | 8.49% | 17.01 : 1 |
| 8-high | 13,171,200 | 9.85% | 18.3% | 9.16 : 1 |
| 9-high | 19,174,400 | 14.3% | 32.7% | 5.98 : 1 |
| 10-high | 23,675,904 | 17.7% | 50.4% | 4.65 : 1 |
| Jack-high | 24,837,120 | 18.6% | 68.9% | 4.39 : 1 |
| Queen-high | 21,457,920 | 16.0% | 85.0% | 5.23 : 1 |
| King-high | 13,939,200 | 10.4% | 95.4% | 8.60 : 1 |
| Total | 127,615,488 | 95.4% | 95.4% | 0.05 : 1 |
(The frequencies given are exact; the probabilities and odds are approximate.)
If aces are not low, simply rotate the hand descriptions so that 6-high replaces 5-high for the best hand and ace-high replaces king-high as the worst hand.
Derivation of frequencies for 7-card lowball hands
The following computations show how the above frequencies for 7-card lowball poker hands were determined. To understand these derivations, the reader should be familiar with the basic properties of the binomial coefficientBinomial coefficient
In mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
s and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory)
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
.
To make a low hand of a specific rank four ranks are chosen that are lower than the high rank. Where
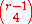
is the highest rank in the hand (numbering jack–king as 11–13), the number of sets of 5 ranks that can make a low hand is:There are then three different ways to choose the remaining two cards that are not used in the low hand. Each of these cases must be considered separately:
7 distinct ranks
In this type of hand the two additional ranks are chosen from the ranks higher than
, so this type of hand can only occur when there are at least two ranks greater than —that is, jack-high or better hands. The suits can be assigned by making 7 independent choices for the suit for each rank, so the number of ways to make a low hand with two distinct higher ranks is: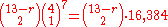6 distinct ranks
In this type of hand there are 6 distinct ranks and one pair. The additional rank is chosen from the ranks higher than
, so this type of hand can only occur when there is at least one rank greater than —that is, queen-high or better hands. One of the 6 ranks is chosen for the pair and two of the four cards in that rank are chosen. The suits for the remaining 5 ranks are assigned by making 5 independent choices for each rank, so the number of ways to make a low hand with one higher ranks and a pair is: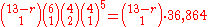5 distinct ranks
There are two ways to choose 5 distinct ranks for seven cards. Either two pair and three unpaired ranks or three of a kind and four unpaired ranks.
- Two pair
- In this type of hand there are 5 distinct ranks and two pair. Two of the 5 ranks are chosen for the pairs and two of the four cards in each rank are chosen. The suits for the remaining 3 ranks are assigned by making 3 independent choices for each rank, so the number of ways to make a low hand with two pair is:
- Three of a kind
- In this type of hand there are 5 distinct ranks and three of a kind. One of the 5 ranks is chosen for the three of a kind and three of the four cards in the rank are chosen. The suits for the remaining 4 ranks are assigned by making 4 independent choices for each rank, so the number of ways to make a low hand with three of a kind is:
Thus there are
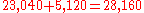ways to make a low hand with five distinct ranks.Derivation
Thus where
is a rank from 5 to jack (11), the total number of -high low hands is:The total number of queen-high low hands is:
The total number of king-high low hands is:
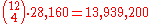
See also
Poker topics:- Poker probability (Texas hold 'em)Poker probability (Texas hold 'em)In poker, the probability of many events can be determined by direct calculation. This article discusses computing probabilities for many commonly occurring events in the game of Texas hold 'em and provides some probabilities and odds for specific situations...
- Poker probability (Omaha)Poker probability (Omaha)In poker, the probability of many events can be determined by direct calculation. This article discusses how to compute the probabilities for many commonly occurring events in the game of Omaha hold 'em and provides some probabilities and odds for specific situations...
Math and probability topics:
- ProbabilityProbabilityProbability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
- OddsOddsThe odds in favor of an event or a proposition are expressed as the ratio of a pair of integers, which is the ratio of the probability that an event will happen to the probability that it will not happen...
- Sample space
- Event (probability theory)Event (probability theory)In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
- Binomial coefficientBinomial coefficientIn mathematics, binomial coefficients are a family of positive integers that occur as coefficients in the binomial theorem. They are indexed by two nonnegative integers; the binomial coefficient indexed by n and k is usually written \tbinom nk , and it is the coefficient of the x k term in...
- CombinationCombinationIn mathematics a combination is a way of selecting several things out of a larger group, where order does not matter. In smaller cases it is possible to count the number of combinations...
- PermutationPermutationIn mathematics, the notion of permutation is used with several slightly different meanings, all related to the act of permuting objects or values. Informally, a permutation of a set of objects is an arrangement of those objects into a particular order...
- Combinatorial game theoryCombinatorial game theoryCombinatorial game theory is a branch of applied mathematics and theoretical computer science that studies sequential games with perfect information, that is, two-player games which have a position in which the players take turns changing in defined ways or moves to achieve a defined winning...
- Game complexityGame complexityCombinatorial game theory has several ways of measuring game complexity. This article describes five of them: state-space complexity, game tree size, decision complexity, game-tree complexity, and computational complexity.-Measures of game complexity:...
- Set theorySet theorySet theory is the branch of mathematics that studies sets, which are collections of objects. Although any type of object can be collected into a set, set theory is applied most often to objects that are relevant to mathematics...
- Gaming mathematicsGaming mathematicsGaming mathematics, also referred to as the mathematics of gambling, is a collection of probability applications encountered in games of chance and can be included in applied mathematics...
External links
- Brian Alspach's mathematics and poker page
- MathWorld: Poker
- Poker probabilities including conditional calculations
- Numerous poker probability tables
- 5, 6, and 7 card poker probabilities
- Poker Odds for Dummies
- The 7,462 and 4,824 equivalence classes
- Preflop, After Flop and Chance of Making Hand Odds
- Odds and Outs probability table
- Poker probability calculator 5, 6 and 7 cards