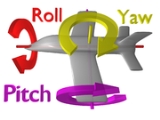
Rigid body - AbsoluteAstronomy.com

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

, a rigid body is an idealization of a solid body

Physical body

In physics, a physical body or physical object is a collection of masses, taken to be one...

of finite size in which deformation is neglected. In other words, the distance

Distance

Distance is a numerical description of how far apart objects are. In physics or everyday discussion, distance may refer to a physical length, or an estimation based on other criteria . In mathematics, a distance function or metric is a generalization of the concept of physical distance...

between any two given point

Point (geometry)

In geometry, topology and related branches of mathematics a spatial point is a primitive notion upon which other concepts may be defined. In geometry, points are zero-dimensional; i.e., they do not have volume, area, length, or any other higher-dimensional analogue. In branches of mathematics...

s of a rigid body remains constant in time regardless of external force

Force

In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...

s exerted on it. Even though such an object cannot physically exist due to relativity

Special relativity

Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...

, objects can normally be assumed to be perfectly rigid if they are not moving near the speed of light

Speed of light

The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...

.

In classical mechanics a rigid body is usually considered as a continuous
mass distribution, while in quantum mechanics

Quantum mechanics

Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...

a rigid body is usually thought of as
a collection of point masses. For instance, in quantum mechanics molecules (consisting of the point masses: electrons and nuclei) are often seen as rigid bodies (see classification of molecules as rigid rotors).

Linear and angular position

The position of a rigid body is the position of all the particles of which it is composed. To simplify the description of this position, we exploit the property that the body is rigid, namely that all its particles maintain the same distance relative to each other. If the body is rigid, it is sufficient to describe the position of at least three non-collinear particles. This makes it possible to reconstruct the position of all the other particles, provided that their time-invariant position relative to the three selected particles is known. However, typically a different, mathematically more convenient, but equivalent approach is used. The position of the whole body is represented by:

the linear position or position of the body, namely the position of one of the particles of the body, specifically chosen as a reference point (typically coinciding with the center of mass
Center of mass
In physics, the center of mass or barycenter of a system is the average location of all of its mass. In the case of a rigid body, the position of the center of mass is fixed in relation to the body...

or centroid
Centroid
In geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...

of the body), together with
the angular position
Orientation (geometry)
In geometry the orientation, angular position, or attitude of an object such as a line, plane or rigid body is part of the description of how it is placed in the space it is in....

(also known as orientation, or attitude) of the body.

Thus, the position of a rigid body has two components: linear and angular, respectively. The same is true for other kinematic

Kinematics

Kinematics is the branch of classical mechanics that describes the motion of bodies and systems without consideration of the forces that cause the motion....

and kinetic

Dynamics (mechanics)

In the field of physics, the study of the causes of motion and changes in motion is dynamics. In other words the study of forces and why objects are in motion. Dynamics includes the study of the effect of torques on motion...

quantities describing the motion of a rigid body, such as linear and angular velocity

Velocity

In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...

, acceleration

Acceleration

In physics, acceleration is the rate of change of velocity with time. In one dimension, acceleration is the rate at which something speeds up or slows down. However, since velocity is a vector, acceleration describes the rate of change of both the magnitude and the direction of velocity. ...

, momentum

Momentum

In classical mechanics, linear momentum or translational momentum is the product of the mass and velocity of an object...

, impulse, and kinetic energy

Kinetic energy

The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...

.

The linear position can be represented by a vector with its tail at an arbitrary reference point in space (the origin of a chosen coordinate system

Coordinate system

In geometry, a coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point or other geometric element. The order of the coordinates is significant and they are sometimes identified by their position in an ordered tuple and sometimes by...

) and its tip at an arbitrary point of interest on the rigid body, typically coinciding with its center of mass

Center of mass

In physics, the center of mass or barycenter of a system is the average location of all of its mass. In the case of a rigid body, the position of the center of mass is fixed in relation to the body...

or centroid

Centroid

In geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...

. This reference point may define the origin of a coordinate system

Coordinate system

fixed to the body.

There are several ways to numerically describe the orientation

Orientation (geometry)

In geometry the orientation, angular position, or attitude of an object such as a line, plane or rigid body is part of the description of how it is placed in the space it is in....

of a rigid body, including a set of three Euler angles

Euler angles

The Euler angles are three angles introduced by Leonhard Euler to describe the orientation of a rigid body. To describe such an orientation in 3-dimensional Euclidean space three parameters are required...

, a quaternion

Quaternion

In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...

, or a direction cosine matrix (also referred to as a rotation matrix). All these methods actually define the orientation of a basis set

Basis set

Basis set can refer to:* Basis * Basis set...

(or coordinate system

Coordinate system

) which has a fixed orientation relative to the body (i.e. rotates together with the body), relative to another basis set (or coordinate system), from which the motion of the rigid body is observed. For instance, a basis set with fixed orientation relative to an airplane can be defined as a set of three orthogonal unit vectors b₁, b₂, b₃, such that b₁ is parallel to the chord line of the wing and directed forward, b₂ is normal to the plane of symmetry and directed rightward, and b₃ is given by the cross product

.

In general, when a rigid body moves, both its position and orientation vary with time. In the kinematic sense, these changes are referred to as translation
Translation (physics)
In physics, translation is movement that changes the position of an object, as opposed to rotation. For example, according to Whittaker:...

and rotation
Rotation
A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...

, respectively. Indeed, the position of a rigid body can be viewed as a hypothetic translation and rotation (roto-translation) of the body starting from a hypothetic reference position (not necessarily coinciding with a position actually taken by the body during its motion).

Linear and angular velocity

Velocity

(also called linear velocity) and angular velocity

Angular velocity

In physics, the angular velocity is a vector quantity which specifies the angular speed of an object and the axis about which the object is rotating. The SI unit of angular velocity is radians per second, although it may be measured in other units such as degrees per second, revolutions per...

are measured with respect to a frame of reference

Frame of reference

A frame of reference in physics, may refer to a coordinate system or set of axes within which to measure the position, orientation, and other properties of objects in it, or it may refer to an observational reference frame tied to the state of motion of an observer.It may also refer to both an...

.

The linear velocity
Velocity
In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...

of a rigid body is a vector quantity, equal to the time rate of change

Time derivative

A time derivative is a derivative of a function with respect to time, usually interpreted as the rate of change of the value of the function. The variable denoting time is usually written as t\,.-Notation:...

of its linear position. Thus, it is the velocity of a reference point fixed to the body. During purely translational motion (motion with no rotation), all points on a rigid body move with the same velocity

Velocity

. However, when motion

Motion (physics)

In physics, motion is a change in position of an object with respect to time. Change in action is the result of an unbalanced force. Motion is typically described in terms of velocity, acceleration, displacement and time . An object's velocity cannot change unless it is acted upon by a force, as...

involves rotation, the instantaneous velocity of any two points on the body will generally not be the same. Two points of a rotating body will have the same instantaneous velocity only if they happen to lay on an axis parallel to the instantaneous axis of rotation.

Angular velocity
Angular velocity
In physics, the angular velocity is a vector quantity which specifies the angular speed of an object and the axis about which the object is rotating. The SI unit of angular velocity is radians per second, although it may be measured in other units such as degrees per second, revolutions per...

is a vector quantity that describes the angular speed at which the orientation of the rigid body is changing and the instantaneous axis about which it is rotating (the existence of this instantaneous axis is guaranteed by the Euler's rotation theorem

Euler's rotation theorem

In geometry, Euler's rotation theorem states that, in three-dimensional space, any displacement of a rigid body such that a point on the rigid body remains fixed, is equivalent to a single rotation about some axis that runs through the fixed point. It also means that the composition of two...

). All points on a rigid body experience the same angular velocity

Angular velocity

at all times. During purely rotational motion, all points on the body change position except for those lying on the instantaneous axis of rotation. The relationship between orientation and angular velocity is not directly analogous to the relationship between position and velocity. Angular velocity is not the time rate of change

Time derivative

of orientation, because there is no such concept as an orientation vector that can be differentiated

Derivative

In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...

to obtain the angular velocity.

Addition theorem for angular velocity

The angular velocity of a rigid body B in a reference frame N is equal to the sum of the angular velocity of a rigid body D in N and the angular velocity of B with respect D:

.

In this case, rigid bodies and reference frames are indistinguishable and completely interchangeable.

Addition theorem for position

For any set of three points P, Q, and R, the position vector from P to R is the sum of the position vector from P to Q and the position vector from Q to R:

Mathematical definition of velocity

The velocity of point P in reference frame N is defined using the time derivative

Time derivative

in N of the position vector from O to P:

where O is any arbitrary point fixed in reference frame N, and the N to the left of the d/dt operator indicates that the derivative is taken in reference frame N. The result is independent of the selection of O so long as O is fixed in N.

Mathematical definition of acceleration

The acceleration of point P in reference frame N is defined using the time derivative

Time derivative

in N of its velocity:

Velocity of two points fixed on a rigid body

For two points P and Q that are fixed on a rigid body B, where B has an angular velocity

in the reference frame N, the velocity of Q in N can be expressed as a function of the velocity of P in N:

Acceleration of two points fixed on a rigid body

By differentiating the equation for the Velocity of two points fixed on a rigid body in N with respect to time, the acceleration in reference frame N of a point Q fixed on a rigid body B can be expressed as

where

is the angular acceleration of B in the reference frame N.

Velocity of one point moving on a rigid body

If the point R is moving in rigid body B while B moves in reference frame N, then the velocity of R in N is

.

where Q is the point fixed in B that is instantaneously coincident with R at the instant of interest. This relation is often combined with the relation for the Velocity of two points fixed on a rigid body.

Acceleration of one point moving on a rigid body

The acceleration in reference frame N of the point R moving in body B while B is moving in frame N is given by

where Q is the point fixed in B that instantaneously coincident with R at the instant of interest. This equation is often combined with Acceleration of two points fixed on a rigid body.

Other quantities

If C is the origin of a local coordinate system

Coordinate system

L, attached to the body,

the spatial or twist acceleration of a rigid body is defined as the spatial acceleration
Spatial acceleration
In physics the study of rigid body motion provides for several ways of defining the acceleration state of a rigid body. The classical definition of acceleration entails following a single particle/point along the rigid body and observing its changes of velocity...

of C (as opposed to material acceleration above);

where

represents the position of the point/particle with respect to the reference point of the body in terms of the local coordinate system L (the rigidity of the body means that this does not depend on time)
is the orientation
Orientation (rigid body)
In geometry the orientation, angular position, or attitude of an object such as a line, plane or rigid body is part of the description of how it is placed in the space it is in....

matrix, an orthogonal matrix
Orthogonal matrix
In linear algebra, an orthogonal matrix , is a square matrix with real entries whose columns and rows are orthogonal unit vectors ....

with determinant 1, representing the orientation
Orientation (rigid body)
In geometry the orientation, angular position, or attitude of an object such as a line, plane or rigid body is part of the description of how it is placed in the space it is in....

(angular position) of the local coordinate system L, with respect to the arbitrary reference orientation of another coordinate system G. Think of this matrix as three orthogonal unit vectors, one in each column, which define the orientation of the axes of L with respect to G.
represents the angular velocity
Angular velocity
In physics, the angular velocity is a vector quantity which specifies the angular speed of an object and the axis about which the object is rotating. The SI unit of angular velocity is radians per second, although it may be measured in other units such as degrees per second, revolutions per...

of the rigid body
represents the total velocity of the point/particle
represents the total acceleration of the point/particle
represents the angular acceleration
Angular acceleration
Angular acceleration is the rate of change of angular velocity over time. In SI units, it is measured in radians per second squared , and is usually denoted by the Greek letter alpha .- Mathematical definition :...

of the rigid body
represents the spatial acceleration
Spatial acceleration
In physics the study of rigid body motion provides for several ways of defining the acceleration state of a rigid body. The classical definition of acceleration entails following a single particle/point along the rigid body and observing its changes of velocity...

of the point/particle
represents the spatial acceleration
Spatial acceleration
In physics the study of rigid body motion provides for several ways of defining the acceleration state of a rigid body. The classical definition of acceleration entails following a single particle/point along the rigid body and observing its changes of velocity...

of the rigid body (i.e. the spatial acceleration of the origin of L)

In 2D the angular velocity is a scalar, and matrix A(t) simply represents a rotation in the xy-plane by an angle which is the integral of the angular velocity over time.

Vehicle

Vehicle

A vehicle is a device that is designed or used to transport people or cargo. Most often vehicles are manufactured, such as bicycles, cars, motorcycles, trains, ships, boats, and aircraft....

s, walking people, etc. usually rotate according to changes in the direction of the velocity: they move forward with respect to their own orientation. Then, if the body follows a closed orbit in a plane, the angular velocity integrated over a time interval in which the orbit is completed once, is an integer times 360°. This integer is the winding number

Winding number

In mathematics, the winding number of a closed curve in the plane around a given point is an integer representing the total number of times that curve travels counterclockwise around the point...

with respect to the origin of the velocity. Compare the amount of rotation associated with the vertices of a polygon.

Kinetics

Any point that is rigidly connected to the body can be used as reference point (origin of coordinate system L) to describe the linear motion of the body (the linear position, velocity and acceleration vectors depend on the choice).

However, depending on the application, a convenient choice may be:

the center of mass
Center of mass
In physics, the center of mass or barycenter of a system is the average location of all of its mass. In the case of a rigid body, the position of the center of mass is fixed in relation to the body...

of the whole system, which generally has the simplest motion for a body moving freely in space;
a point such that the translational motion is zero or simplified, e.g. on an axle
Axle
An axle is a central shaft for a rotating wheel or gear. On wheeled vehicles, the axle may be fixed to the wheels, rotating with them, or fixed to its surroundings, with the wheels rotating around the axle. In the former case, bearings or bushings are provided at the mounting points where the axle...

or hinge
Hinge
A hinge is a type of bearing that connects two solid objects, typically allowing only a limited angle of rotation between them. Two objects connected by an ideal hinge rotate relative to each other about a fixed axis of rotation. Hinges may be made of flexible material or of moving components...

, at the center of a ball and socket joint
Ball and socket joint
A ball and socket joint is a joint in which the distal bone is capable of motion around an indefinite number of axes, which have one common center...

, etc.

When the center of mass is used as reference point:

The (linear) momentum
Momentum
In classical mechanics, linear momentum or translational momentum is the product of the mass and velocity of an object...

is independent of the rotational motion. At any time it is equal to the total mass of the rigid body times the translational velocity.
The angular momentum
Angular momentum
In physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...

with respect to the center of mass is the same as without translation: at any time it is equal to the inertia tensor
Moment of inertia
In classical mechanics, moment of inertia, also called mass moment of inertia, rotational inertia, polar moment of inertia of mass, or the angular mass, is a measure of an object's resistance to changes to its rotation. It is the inertia of a rotating body with respect to its rotation...

times the angular velocity. When the angular velocity is expressed with respect to a coordinate system coinciding with the principal axes of the body, each component of the angular momentum is a product of a moment of inertia (a principal value of the inertia tensor) times the corresponding component of the angular velocity; the torque
Torque
Torque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....

is the inertia tensor times the angular acceleration
Angular acceleration
Angular acceleration is the rate of change of angular velocity over time. In SI units, it is measured in radians per second squared , and is usually denoted by the Greek letter alpha .- Mathematical definition :...

.
Possible motions in the absence of external forces are translation with constant velocity, steady rotation about a fixed principal axis, and also torque-free precession
Precession
Precession is a change in the orientation of the rotation axis of a rotating body. It can be defined as a change in direction of the rotation axis in which the second Euler angle is constant...

.
The net external force on the rigid body is always equal to the total mass times the translational acceleration (i.e., Newton's second law holds for the translational motion, even when the net external torque is nonzero, and/or the body rotates).
The total kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...

is simply the sum of translational and rotational energy
Rotational energy
The rotational energy or angular kinetic energy is the kinetic energy due to the rotation of an object and is part of its total kinetic energy...

.

Geometry

Two rigid bodies are said to be different (not copies) if there is no proper rotation from one to the other.
A rigid body is called chiral

Chirality (mathematics)

In geometry, a figure is chiral if it is not identical to its mirror image, or, more precisely, if it cannot be mapped to its mirror image by rotations and translations alone. For example, a right shoe is different from a left shoe, and clockwise is different from counterclockwise.A chiral object...

if its mirror image

Mirror image

A mirror image is a reflected duplication of an object that appears identical but reversed. As an optical effect it results from reflection off of substances such as a mirror or water. It is also a concept in geometry and can be used as a conceptualization process for 3-D structures...

is different in that sense, i.e., if it has either no symmetry

Symmetry

Symmetry generally conveys two primary meanings. The first is an imprecise sense of harmonious or aesthetically pleasing proportionality and balance; such that it reflects beauty or perfection...

or its symmetry group

Symmetry group

The symmetry group of an object is the group of all isometries under which it is invariant with composition as the operation...

contains only proper rotations. In the opposite case an object is called achiral: the mirror image is a copy, not a different object. Such an object may have a symmetry plane, but not necessarily: there may also be a plane of reflection with respect to which the image of the object is a rotated version. The latter applies for S_2n

Point groups in three dimensions

In geometry, a point group in three dimensions is an isometry group in three dimensions that leaves the origin fixed, or correspondingly, an isometry group of a sphere. It is a subgroup of the orthogonal group O, the group of all isometries that leave the origin fixed, or correspondingly, the group...

, of which the case n = 1 is inversion symmetry.

For a (rigid) rectangular transparent sheet, inversion symmetry corresponds to having on one side an image without rotational symmetry and on the other side an image such that what shines through is the image at the top side, upside down. We can distinguish two cases:

the sheet surface with the image is not symmetric - in this case the two sides are different, but the mirror image of the object is the same, after a rotation by 180° about the axis perpendicular to the mirror plane.
the sheet surface with the image has a symmetry axis - in this case the two sides are the same, and the mirror image of the object is also the same, again after a rotation by 180° about the axis perpendicular to the mirror plane.

A sheet with a through and through

Through and through

Through and through describes a situation where an object, real or imaginary, passes completely through another object, also real or imaginary...

image is achiral. We can distinguish again two cases:

the sheet surface with the image has no symmetry axis - the two sides are different
the sheet surface with the image has a symmetry axis - the two sides are the same

Configuration space

The configuration space

Configuration space

- Configuration space in physics :In classical mechanics, the configuration space is the space of possible positions that a physical system may attain, possibly subject to external constraints...

of a rigid body with one point fixed (i.e., a body with zero translational motion) is given by the underlying manifold

Manifold

In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....

of the rotation group

Rotation group

In mechanics and geometry, the rotation group is the group of all rotations about the origin of three-dimensional Euclidean space R3 under the operation of composition. By definition, a rotation about the origin is a linear transformation that preserves length of vectors and preserves orientation ...

SO(3). The configuration space of a nonfixed (with non-zero translational motion) rigid body is E⁺(3), the subgroup of direct isometries of the Euclidean group

Euclidean group

In mathematics, the Euclidean group E, sometimes called ISO or similar, is the symmetry group of n-dimensional Euclidean space...

in three dimensions (combinations of translations

Translation (geometry)

In Euclidean geometry, a translation moves every point a constant distance in a specified direction. A translation can be described as a rigid motion, other rigid motions include rotations and reflections. A translation can also be interpreted as the addition of a constant vector to every point, or...

and rotation

Rotation

A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...

s).

Linear and angular position

Linear and angular velocity

Addition theorem for angular velocity

Addition theorem for position

Mathematical definition of velocity

Mathematical definition of acceleration

Velocity of two points fixed on a rigid body

Acceleration of two points fixed on a rigid body

Velocity of one point moving on a rigid body

Acceleration of one point moving on a rigid body

Other quantities

Kinetics

Geometry

Configuration space

See also