Yuktibhasa
Encyclopedia
Yuktibhāṣā also known as Gaṇitanyāyasaṅgraha ("Compendium of astronomical rationale"), is a major treatise
on mathematics
and astronomy
, written by India
n astronomer Jyesthadeva of the Kerala school of mathematics in about AD 1530. The treatise is a consolidation of the discoveries by Madhava of Sangamagrama
, Nilakantha Somayaji
, Parameswara, Jyeshtadeva
, Achyuta Pisharati
and other astronomer-mathematicians of the Kerala school. Yuktibhasa is mainly based on Nilakantha's Tantra Samgraha. It is considered an early text on some of the foundations of calculus
and predates those of Europe
an mathematicians such as James Gregory by over a century. However, the treatise was largely unnoticed beyond Kerala
, as the book was written in the local language of Malayalam. However, some have argued that mathematics from Kerala were transmitted to Europe (see Possible transmission of Keralese mathematics to Europe).
The work was unique for its time, since it contained proof
s and derivations of the theorem
s that it presented; something that was not usually done by any Indian mathematicians of that era. Some of its important developments in analysis include: the infinite series expansion of a function, the power series, the Taylor series
, the trigonometric series for sine
, cosine, tangent and arctangent, the second and third order Taylor series approximations of sine
and cosine, the power series of π, π/4, θ, the radius, diameter and circumference, and tests of convergence
.
and Nilakantha
. The text is divided into two parts — the former deals with mathematical analysis
of arithmetic
, algebra
, trigonometry
and geometry
, logistic
s, algebraic problems, fraction
s, Rule of three, Kuttakaram, circle
and disquisition on R-Sine; and the latter about astronomy.
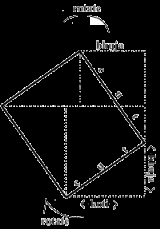
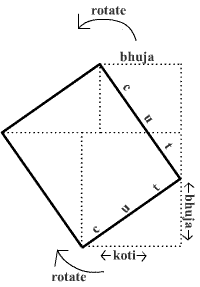As per the old Indian tradition of starting off new chapters with elementary content, the first four chapters of the Yuktibhasa contain elementary mathematics, such as division, proof of Pythagorean theorem
, square root
determination, etc. The radical ideas are not discussed until the sixth chapter on circumference
of a circle
.
Yuktibhasa contains the derivation and proof of the power series for inverse tangent
, discovered by Madhava. In the text, Jyesthadeva describes Madhava's series in the following manner:
This yields
Treatise
A treatise is a formal and systematic written discourse on some subject, generally longer and treating it in greater depth than an essay, and more concerned with investigating or exposing the principles of the subject.-Noteworthy treatises:...
on mathematics
Indian mathematics
Indian mathematics emerged in the Indian subcontinent from 1200 BCE until the end of the 18th century. In the classical period of Indian mathematics , important contributions were made by scholars like Aryabhata, Brahmagupta, and Bhaskara II. The decimal number system in use today was first...
and astronomy
Hindu astronomy
Historical Indian astronomy develops as a discipline of Vedanga or one of the "auxiliary disciplines" associated with the study of the Vedas.The oldest extant text of astronomy is the treatise by Lagadha, dated to the Mauryan era ....
, written by India
India
India , officially the Republic of India , is a country in South Asia. It is the seventh-largest country by geographical area, the second-most populous country with over 1.2 billion people, and the most populous democracy in the world...
n astronomer Jyesthadeva of the Kerala school of mathematics in about AD 1530. The treatise is a consolidation of the discoveries by Madhava of Sangamagrama
Madhava of Sangamagrama
Mādhava of Sañgamāgrama was a prominent Kerala mathematician-astronomer from the town of Irińńālakkuţa near Cochin, Kerala, India. He is considered the founder of the Kerala School of Astronomy and Mathematics...
, Nilakantha Somayaji
Nilakantha Somayaji
Kelallur Nilakantha Somayaji was a major mathematician and astronomer of the Kerala school of astronomy and mathematics. One of his most influential works was the comprehensive astronomical treatise Tantrasamgraha completed in 1501...
, Parameswara, Jyeshtadeva
Jyeshtadeva
Jyeṣṭhadeva was an astronomer-mathematician of the Kerala school of astronomy and mathematics founded by Sangamagrama Madhava . He is best known as the author of Yuktibhāṣā,...
, Achyuta Pisharati
Achyuta Pisharati
Acyuta Piṣāraṭi was an Sanskrit grammarian, astrologer, astronomer and mathematician who studied under Jyeṣṭhadeva and was a member of Mādhava of Sañgamāgrama's Kerala school of astronomy and mathematics...
and other astronomer-mathematicians of the Kerala school. Yuktibhasa is mainly based on Nilakantha's Tantra Samgraha. It is considered an early text on some of the foundations of calculus
Calculus
Calculus is a branch of mathematics focused on limits, functions, derivatives, integrals, and infinite series. This subject constitutes a major part of modern mathematics education. It has two major branches, differential calculus and integral calculus, which are related by the fundamental theorem...
and predates those of Europe
Europe
Europe is, by convention, one of the world's seven continents. Comprising the westernmost peninsula of Eurasia, Europe is generally 'divided' from Asia to its east by the watershed divides of the Ural and Caucasus Mountains, the Ural River, the Caspian and Black Seas, and the waterways connecting...
an mathematicians such as James Gregory by over a century. However, the treatise was largely unnoticed beyond Kerala
Kerala
or Keralam is an Indian state located on the Malabar coast of south-west India. It was created on 1 November 1956 by the States Reorganisation Act by combining various Malayalam speaking regions....
, as the book was written in the local language of Malayalam. However, some have argued that mathematics from Kerala were transmitted to Europe (see Possible transmission of Keralese mathematics to Europe).
The work was unique for its time, since it contained proof
Mathematical proof
In mathematics, a proof is a convincing demonstration that some mathematical statement is necessarily true. Proofs are obtained from deductive reasoning, rather than from inductive or empirical arguments. That is, a proof must demonstrate that a statement is true in all cases, without a single...
s and derivations of the theorem
Theorem
In mathematics, a theorem is a statement that has been proven on the basis of previously established statements, such as other theorems, and previously accepted statements, such as axioms...
s that it presented; something that was not usually done by any Indian mathematicians of that era. Some of its important developments in analysis include: the infinite series expansion of a function, the power series, the Taylor series
Taylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
, the trigonometric series for sine
Sine
In mathematics, the sine function is a function of an angle. In a right triangle, sine gives the ratio of the length of the side opposite to an angle to the length of the hypotenuse.Sine is usually listed first amongst the trigonometric functions....
, cosine, tangent and arctangent, the second and third order Taylor series approximations of sine
Sine
In mathematics, the sine function is a function of an angle. In a right triangle, sine gives the ratio of the length of the side opposite to an angle to the length of the hypotenuse.Sine is usually listed first amongst the trigonometric functions....
and cosine, the power series of π, π/4, θ, the radius, diameter and circumference, and tests of convergence
Integral test for convergence
In mathematics, the integral test for convergence is a method used to test infinite series of non-negative terms for convergence. An early form of the test of convergence was developed in India by Madhava in the 14th century, and by his followers at the Kerala School...
.
Contents
Yuktibhasa contains most of the developments of earlier Kerala School mathematicians, particularly MadhavaMadhava of Sangamagrama
Mādhava of Sañgamāgrama was a prominent Kerala mathematician-astronomer from the town of Irińńālakkuţa near Cochin, Kerala, India. He is considered the founder of the Kerala School of Astronomy and Mathematics...
and Nilakantha
Nilakantha Somayaji
Kelallur Nilakantha Somayaji was a major mathematician and astronomer of the Kerala school of astronomy and mathematics. One of his most influential works was the comprehensive astronomical treatise Tantrasamgraha completed in 1501...
. The text is divided into two parts — the former deals with mathematical analysis
Mathematical analysis
Mathematical analysis, which mathematicians refer to simply as analysis, has its beginnings in the rigorous formulation of infinitesimal calculus. It is a branch of pure mathematics that includes the theories of differentiation, integration and measure, limits, infinite series, and analytic functions...
of arithmetic
Arithmetic
Arithmetic or arithmetics is the oldest and most elementary branch of mathematics, used by almost everyone, for tasks ranging from simple day-to-day counting to advanced science and business calculations. It involves the study of quantity, especially as the result of combining numbers...
, algebra
Algebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
, trigonometry
Trigonometry
Trigonometry is a branch of mathematics that studies triangles and the relationships between their sides and the angles between these sides. Trigonometry defines the trigonometric functions, which describe those relationships and have applicability to cyclical phenomena, such as waves...
and geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, logistic
Logistic
Logistic may refer to:* Logistics, the management of resources and their distributions** Logistic engineering, the scientific study of logistics** Military logistics, the study of logistics at the service of military units and operations...
s, algebraic problems, fraction
Fraction (mathematics)
A fraction represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, we specify how many parts of a certain size there are, for example, one-half, five-eighths and three-quarters.A common or "vulgar" fraction, such as 1/2, 5/8, 3/4, etc., consists...
s, Rule of three, Kuttakaram, circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
and disquisition on R-Sine; and the latter about astronomy.
Mathematics
Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a right triangle...
, square root
Square root
In mathematics, a square root of a number x is a number r such that r2 = x, or, in other words, a number r whose square is x...
determination, etc. The radical ideas are not discussed until the sixth chapter on circumference
Circumference
The circumference is the distance around a closed curve. Circumference is a special perimeter.-Circumference of a circle:The circumference of a circle is the length around it....
of a circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
.
Yuktibhasa contains the derivation and proof of the power series for inverse tangent
Inverse trigonometric function
In mathematics, the inverse trigonometric functions are the inverse functions of the trigonometric functions with suitably restricted domains .The notations sin−1, cos−1, etc...
, discovered by Madhava. In the text, Jyesthadeva describes Madhava's series in the following manner:
This yields
-
which further yields the theorem
attributed to James GregoryJames Gregory (astronomer and mathematician)James Gregory FRS was a Scottish mathematician and astronomer. He described an early practical design for the reflecting telescope – the Gregorian telescope – and made advances in trigonometry, discovering infinite series representations for several trigonometric functions.- Biography :The...
, who discovered it three centuries after Madhava.
The text also elucidates Madhava's infinite seriesSeries (mathematics)A series is the sum of the terms of a sequence. Finite sequences and series have defined first and last terms, whereas infinite sequences and series continue indefinitely....
expansion of π:
which he obtained from the power series expansion of the arc-tangent function.
Using a rational approximation of this series, he gave values of the number π as 3.14159265359 - correct to 11 decimals; and as 3.1415926535898 - correct to 13 decimals. These were the most accurate approximations of π after almost a thousand years.
The text describes that he gave two methods for computing the value of π.
- One of these methods is to obtain a rapidly converging series by transforming the original infinite series of π. By doing so, he obtained the infinite series
and used the first 21 terms to compute an approximation of π correct to 11 decimal places as 3.14159265359.
- The other method was to add a remainder term to the original series of π. The remainder term was used
in the infinite series expansion of
to improve the approximation of π to 13 decimal places of accuracy when n = 76.
Apart from these, the Yukthibhasa contains many elementaryElementary mathematicsElementary mathematics consists of mathematics topics frequently taught at the primary or secondary school levels. The most basic topics in elementary mathematics are arithmetic and geometry...
, and complex mathematics, including,- Proof for the expansion of the sineSineIn mathematics, the sine function is a function of an angle. In a right triangle, sine gives the ratio of the length of the side opposite to an angle to the length of the hypotenuse.Sine is usually listed first amongst the trigonometric functions....
and cosine functions. - Integer solutions of systems of first degree equations (solved using a system known as kuttakaram)
- Rules for finding the sines and the cosines of the sum and difference of two anglesAnglesThe Angles is a modern English term for a Germanic people who took their name from the ancestral cultural region of Angeln, a district located in Schleswig-Holstein, Germany...
. - The earliest statement of and the Taylor seriesTaylor seriesIn mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
(only some for some functions). - Geometric derivations of series.
- Tests of convergence (often attributed to CauchyAugustin Louis CauchyBaron Augustin-Louis Cauchy was a French mathematician who was an early pioneer of analysis. He started the project of formulating and proving the theorems of infinitesimal calculus in a rigorous manner, rejecting the heuristic principle of the generality of algebra exploited by earlier authors...
) - Fundamentals of calculus: differentiationDerivativeIn calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
, term by term integrationIntegralIntegration is an important concept in mathematics and, together with its inverse, differentiation, is one of the two main operations in calculus...
, iterative methodIterative methodIn computational mathematics, an iterative method is a mathematical procedure that generates a sequence of improving approximate solutions for a class of problems. A specific implementation of an iterative method, including the termination criteria, is an algorithm of the iterative method...
s for solutions of non-linearNonlinearityIn mathematics, a nonlinear system is one that does not satisfy the superposition principle, or one whose output is not directly proportional to its input; a linear system fulfills these conditions. In other words, a nonlinear system is any problem where the variable to be solved for cannot be...
equations, and the theory that the area under a curve is its integral.
Most of these results were long before their European counterparts, to whom credit was traditionally attributed.
Astronomy
Chapters seven to seventeen of the text deals essentially with subjects of astronomy, viz. Planetary orbitPlanetary orbitIn physics, an orbit is the gravitationally curved path of an object around a point in space, for example the orbit of a planet around the center of a star system, such as the Solar System...
, Celestial sphereCelestial sphereIn astronomy and navigation, the celestial sphere is an imaginary sphere of arbitrarily large radius, concentric with the Earth and rotating upon the same axis. All objects in the sky can be thought of as projected upon the celestial sphere. Projected upward from Earth's equator and poles are the...
, ascensionRight ascensionRight ascension is the astronomical term for one of the two coordinates of a point on the celestial sphere when using the equatorial coordinate system. The other coordinate is the declination.-Explanation:...
, declinationDeclinationIn astronomy, declination is one of the two coordinates of the equatorial coordinate system, the other being either right ascension or hour angle. Declination in astronomy is comparable to geographic latitude, but projected onto the celestial sphere. Declination is measured in degrees north and...
, directions and shadows, spherical triangleSpherical trigonometrySpherical trigonometry is a branch of spherical geometry which deals with polygons on the sphere and the relationships between the sides and the angles...
s, ellipseEllipseIn geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
s and parallaxParallaxParallax is a displacement or difference in the apparent position of an object viewed along two different lines of sight, and is measured by the angle or semi-angle of inclination between those two lines. The term is derived from the Greek παράλλαξις , meaning "alteration"...
correction. The planetary theory described in the book is similar to that later adopted by Danish astronomer Tycho BraheTycho BraheTycho Brahe , born Tyge Ottesen Brahe, was a Danish nobleman known for his accurate and comprehensive astronomical and planetary observations...
.
Modern edition of Yuktibhasa
The importance of Yuktibhasa was brought to the attention of modern scholarship by C.M. Whish in 1834 through a paper published in the Transactions of the Royal Asiatic Society of Great Britain and Ireland. However, an edition of the mathematics part of the text (along with notes in Malayalam) was published only in 1948 by Ramavarma Thampuran and Akhileswara Aiyar. For the first time, a critical edition of the entire Malayalam text, along with English translation and detailed explanatory notes, has been brought out in two volumes by SpringerSpringer Science+Business Media- Selected publications :* Encyclopaedia of Mathematics* Ergebnisse der Mathematik und ihrer Grenzgebiete * Graduate Texts in Mathematics * Grothendieck's Séminaire de géométrie algébrique...
in 2008.
A third volume presenting a critical edition of the Sanskrit Ganitayuktibhasa has been brought out by the Indian Institute of Advanced Study, Shimla in 2009.
See also
- Ganita-yukti-bhasaGanita-yukti-bhasaGanita-yukti-bhasa is either the title or a part of the title of three different books:*Ganita-yukti-bhasa of Jyesthadeva, published by Springer, is the first critical edition with an English translation of Yuktibhasa, a seminal treatise in the Malayalam language composed in c.1530 CE by...
- Indian mathematicsIndian mathematicsIndian mathematics emerged in the Indian subcontinent from 1200 BCE until the end of the 18th century. In the classical period of Indian mathematics , important contributions were made by scholars like Aryabhata, Brahmagupta, and Bhaskara II. The decimal number system in use today was first...
- Kerala SchoolKerala SchoolThe Kerala school of astronomy and mathematics was a school of mathematics and astronomy founded by Madhava of Sangamagrama in Kerala, India, which included among its members: Parameshvara, Neelakanta Somayaji, Jyeshtadeva, Achyuta Pisharati, Melpathur Narayana Bhattathiri and Achyuta Panikkar...
- Possible transmission of Kerala mathematics to Europe
External links