
Closed and exact differential forms
Encyclopedia
In mathematics
, especially vector calculus and differential topology
, a closed form is a differential form
α whose exterior derivative
is zero (dα = 0), and an exact form is a differential form that is the exterior derivative
of another differential form β. Thus, an exact form is in the image
of d, and a closed form is in the kernel
of d.
For an exact form α, α = dβ for some differential form β of one-lesser degree than α. The form β is called a "potential form" or "primitive" for α. Since d2 = 0, β is not unique, but can be modified by the addition of the differential of a two-step-lower-order form.
Because d2 = 0, any exact form is automatically closed. The question of whether every closed form is exact depends on the topology
of the domain of interest. On a contractible
domain, every closed form is exact by the Poincaré lemma. More general questions of this kind on an arbitrary differentiable manifold
are the subject of de Rham cohomology
, that allows one to obtain purely topological
information using differential methods.
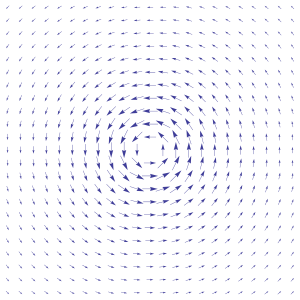 The simplest example of a form which is closed but not exact is the 1-form "dθ" (quotes because it is not the derivative of a globally defined function), defined on the punctured plane
The simplest example of a form which is closed but not exact is the 1-form "dθ" (quotes because it is not the derivative of a globally defined function), defined on the punctured plane  which is locally given as the derivative of the argument - note that argument is locally but not globally defined, since a loop around the origin increases (or decreases, depending on direction) the argument by 2π, which corresponds to the integral:
which is locally given as the derivative of the argument - note that argument is locally but not globally defined, since a loop around the origin increases (or decreases, depending on direction) the argument by 2π, which corresponds to the integral:
and for general paths is known as the winding number
. The differential of the argument is however globally defined (except at the origin), since differentiation only requires local data and different values of the argument differ by a constant, so the derivatives of different local definitions are equal; this line of thought is generalized in the notion of covering spaces.
Explicitly, the form is given as:
which is not defined at the origin. This can be computed from a formula for the argument, most simply via arctan(y/x) (y/x is the slope of the line passing through (x,y), and arctan converts slope to angle), recognizing 1/(x2+y2) as corresponding to the derivative of arctan, which is 1/(x2+1) (these agree on the line y=1). While the differential is correctly computed by symbolically differentiating this expression, this formula is only strictly correct on the halfplane x>0, and properly one must use a correct formula for the argument.
This form generates the de Rham cohomology group meaning that any closed form
meaning that any closed form  is the sum of an exact form
is the sum of an exact form  and a multiple of
and a multiple of 
 where
where  accounts for a non-trivial contour integral around the origin, which is the only obstruction to a closed form on the punctured plane (locally the derivative of a potential function
accounts for a non-trivial contour integral around the origin, which is the only obstruction to a closed form on the punctured plane (locally the derivative of a potential function
) being the derivative of a globally defined function.
of the nineteenth century. In the plane, 0-forms are just functions, and 2-forms are functions times the basic area element dx∧dy, so that it is the 1-forms

that are of real interest. The formula for the exterior derivative
d here is

where the subscripts denote partial derivative
s. Therefore the condition for α to be closed is

In this case if h(x,y) is a function then

The implication from 'exact' to 'closed' is then a consequence of the symmetry of second derivatives
, with respect to x and y.
, or more generally a pseudo-Riemannian manifold
, k-forms correspond to k-vector fields (by duality via the metric), so there is a notion of a vector field corresponding to a closed or exact form.
In 3 dimensions, an exact vector field (thought of as a 1-form) is called a conservative vector field, meaning that it is the derivative (gradient
) of a 0-form (function), called the scalar potential
. A closed vector field (thought of as a 1-form) is one whose derivative (curl) vanishes, and is called an irrotational vector field
.
Thinking of a vector field as a 2-form instead, a closed vector field is one whose derivative (divergence
) vanishes, and is called an incompressible flow
(sometimes solenoidal vector field).
Conservative and incompressible vector fields generalize to n-dimensions (gradient and divergence generalize to n dimensions); curl and hence irrotational does not generalize in this way.
open subset of Rn, any smooth closed p-form α defined on X is exact, for any integer p > 0 (this has content only when p ≤ n).
Contractibility means that there is a homotopy
Ft : X×[0,1] → X that continuously deforms X to a point. Thus every cycle c in X is the boundary of some "cone"; one may take the cone to be the image of c under the homotopy. A dual version of this gives the Poincaré lemma.
More specifically, we associate to X the cylinder X×[0,1]. Identify the top and bottom of the cylinder with the maps j1(x) = (x, 1) and j0(x) = (x, 0) respectively. On the differential forms, the induced maps j1* and j0* are related by a cochain homotopy K:

Let Ωp(X) denote the p-forms on X. The map K: Ωp + 1( X×[0,1] ) → Ωp(X) is the dual of the cylinder map and defined by

where dxp is a monomial p-form with no dt in it. So if F is a homotopy deforming X to a point Q, then

On forms,

Inserting these two equations into the cochain homotopy equation proves the Poincaré lemma.

then one says that ζ and η are cohomologous to each other. Exact forms are sometimes said to be cohomologous to zero. The set of all forms cohomologous to a given form (and thus to each other) is called a de Rham cohomology
class; the general study of such classes is known as cohomology
. It makes no real sense to ask whether a 0-form (smooth function) is exact, since d increases degree by 1; but the clues from topology suggest that only the zero function should be called "exact". The cohomology classes are identified with locally constant functions.
A corollary of the Poincaré lemma is that de Rham cohomology is homotopy-invariant. Non-contractible spaces need not have trivial de Rham cohomology. For instance, on the circle S1, parametrized by t in [0, 1], the closed 1-form dt is not exact.
 produced by a stationary electrical current is important. There one deals with the vector potential
produced by a stationary electrical current is important. There one deals with the vector potential
 of this field. This case corresponds to k=2, and the defining region is the full
of this field. This case corresponds to k=2, and the defining region is the full  The current-density vector is
The current-density vector is  It corresponds to the current two-form
It corresponds to the current two-form

For the magnetic field one has analogous results: it corresponds to the induction two-form
one has analogous results: it corresponds to the induction two-form  and can be derived from the vector potential
and can be derived from the vector potential  , or the corresponding one-form
, or the corresponding one-form  ,
,

Thereby the vector potential corresponds to the potential one-form
corresponds to the potential one-form

The closedness of the magnetic-induction two-form corresponds to the property of the magnetic field that it is source-free: i.e. there are no magnetic monopole
i.e. there are no magnetic monopole
s.
In a special gauge, , this implies for i = 1, 2, 3
, this implies for i = 1, 2, 3

(Here is a constant, the magnetic vacuum permeability.)
is a constant, the magnetic vacuum permeability.)
This equation is remarkable, because it corresponds completely to a well-known formula for the electrical field , namely for the electrostatic Coulomb potential
, namely for the electrostatic Coulomb potential  of a charge density
of a charge density  . At this place one can already guess that
. At this place one can already guess that
can be unified to quantities with six rsp. four nontrivial components, which is the basis of the relativistic invariance of the Maxwell equations.
If the condition of stationarity is left, on the l.h.s. of the above-mentioned equation one must add, in the equations for to the three space coordinates, as a fourth variable also the time t, whereas on the r.h.s., in
to the three space coordinates, as a fourth variable also the time t, whereas on the r.h.s., in  the so-called "retarded time",
the so-called "retarded time",  must be used, i.e. it is added to the argument of the current-density. Finally, as before, one integrates over the three primed space coordinates. (As usual
must be used, i.e. it is added to the argument of the current-density. Finally, as before, one integrates over the three primed space coordinates. (As usual
c is the vacuum velocity of light.)
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, especially vector calculus and differential topology
Differential topology
In mathematics, differential topology is the field dealing with differentiable functions on differentiable manifolds. It is closely related to differential geometry and together they make up the geometric theory of differentiable manifolds.- Description :...
, a closed form is a differential form
Differential form
In the mathematical fields of differential geometry and tensor calculus, differential forms are an approach to multivariable calculus that is independent of coordinates. Differential forms provide a better definition for integrands in calculus...
α whose exterior derivative
Exterior derivative
In differential geometry, the exterior derivative extends the concept of the differential of a function, which is a 1-form, to differential forms of higher degree. Its current form was invented by Élie Cartan....
is zero (dα = 0), and an exact form is a differential form that is the exterior derivative
Exterior derivative
In differential geometry, the exterior derivative extends the concept of the differential of a function, which is a 1-form, to differential forms of higher degree. Its current form was invented by Élie Cartan....
of another differential form β. Thus, an exact form is in the image
Image (mathematics)
In mathematics, an image is the subset of a function's codomain which is the output of the function on a subset of its domain. Precisely, evaluating the function at each element of a subset X of the domain produces a set called the image of X under or through the function...
of d, and a closed form is in the kernel
Kernel (algebra)
In the various branches of mathematics that fall under the heading of abstract algebra, the kernel of a homomorphism measures the degree to which the homomorphism fails to be injective. An important special case is the kernel of a matrix, also called the null space.The definition of kernel takes...
of d.
For an exact form α, α = dβ for some differential form β of one-lesser degree than α. The form β is called a "potential form" or "primitive" for α. Since d2 = 0, β is not unique, but can be modified by the addition of the differential of a two-step-lower-order form.
Because d2 = 0, any exact form is automatically closed. The question of whether every closed form is exact depends on the topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
of the domain of interest. On a contractible
Contractible space
In mathematics, a topological space X is contractible if the identity map on X is null-homotopic, i.e. if it is homotopic to some constant map. Intuitively, a contractible space is one that can be continuously shrunk to a point....
domain, every closed form is exact by the Poincaré lemma. More general questions of this kind on an arbitrary differentiable manifold
Differentiable manifold
A differentiable manifold is a type of manifold that is locally similar enough to a linear space to allow one to do calculus. Any manifold can be described by a collection of charts, also known as an atlas. One may then apply ideas from calculus while working within the individual charts, since...
are the subject of de Rham cohomology
De Rham cohomology
In mathematics, de Rham cohomology is a tool belonging both to algebraic topology and to differential topology, capable of expressing basic topological information about smooth manifolds in a form particularly adapted to computation and the concrete representation of cohomology classes...
, that allows one to obtain purely topological
Algebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
information using differential methods.
Examples

 which is locally given as the derivative of the argument - note that argument is locally but not globally defined, since a loop around the origin increases (or decreases, depending on direction) the argument by 2π, which corresponds to the integral:
which is locally given as the derivative of the argument - note that argument is locally but not globally defined, since a loop around the origin increases (or decreases, depending on direction) the argument by 2π, which corresponds to the integral:
and for general paths is known as the winding number
Winding number
In mathematics, the winding number of a closed curve in the plane around a given point is an integer representing the total number of times that curve travels counterclockwise around the point...
. The differential of the argument is however globally defined (except at the origin), since differentiation only requires local data and different values of the argument differ by a constant, so the derivatives of different local definitions are equal; this line of thought is generalized in the notion of covering spaces.
Explicitly, the form is given as:

which is not defined at the origin. This can be computed from a formula for the argument, most simply via arctan(y/x) (y/x is the slope of the line passing through (x,y), and arctan converts slope to angle), recognizing 1/(x2+y2) as corresponding to the derivative of arctan, which is 1/(x2+1) (these agree on the line y=1). While the differential is correctly computed by symbolically differentiating this expression, this formula is only strictly correct on the halfplane x>0, and properly one must use a correct formula for the argument.
This form generates the de Rham cohomology group
 meaning that any closed form
meaning that any closed form  is the sum of an exact form
is the sum of an exact form  and a multiple of
and a multiple of 
 where
where  accounts for a non-trivial contour integral around the origin, which is the only obstruction to a closed form on the punctured plane (locally the derivative of a potential function
accounts for a non-trivial contour integral around the origin, which is the only obstruction to a closed form on the punctured plane (locally the derivative of a potential functionPotential function
The term potential function may refer to:* A mathematical function whose values are a physical potential.* The class of functions known as harmonic functions, which are the topic of study in potential theory.* The potential function of a potential game....
) being the derivative of a globally defined function.
Examples in low dimensions
Differential forms in R2 and R3 were well-known in the mathematical physicsMathematical physics
Mathematical physics refers to development of mathematical methods for application to problems in physics. The Journal of Mathematical Physics defines this area as: "the application of mathematics to problems in physics and the development of mathematical methods suitable for such applications and...
of the nineteenth century. In the plane, 0-forms are just functions, and 2-forms are functions times the basic area element dx∧dy, so that it is the 1-forms

that are of real interest. The formula for the exterior derivative
Exterior derivative
In differential geometry, the exterior derivative extends the concept of the differential of a function, which is a 1-form, to differential forms of higher degree. Its current form was invented by Élie Cartan....
d here is

where the subscripts denote partial derivative
Partial derivative
In mathematics, a partial derivative of a function of several variables is its derivative with respect to one of those variables, with the others held constant...
s. Therefore the condition for α to be closed is

In this case if h(x,y) is a function then

The implication from 'exact' to 'closed' is then a consequence of the symmetry of second derivatives
Symmetry of second derivatives
In mathematics, the symmetry of second derivatives refers to the possibility of interchanging the order of taking partial derivatives of a functionfof n variables...
, with respect to x and y.
Vector field analogies
On a Riemannian manifoldRiemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
, or more generally a pseudo-Riemannian manifold
Pseudo-Riemannian manifold
In differential geometry, a pseudo-Riemannian manifold is a generalization of a Riemannian manifold. It is one of many mathematical objects named after Bernhard Riemann. The key difference between a Riemannian manifold and a pseudo-Riemannian manifold is that on a pseudo-Riemannian manifold the...
, k-forms correspond to k-vector fields (by duality via the metric), so there is a notion of a vector field corresponding to a closed or exact form.
In 3 dimensions, an exact vector field (thought of as a 1-form) is called a conservative vector field, meaning that it is the derivative (gradient
Gradient
In vector calculus, the gradient of a scalar field is a vector field that points in the direction of the greatest rate of increase of the scalar field, and whose magnitude is the greatest rate of change....
) of a 0-form (function), called the scalar potential
Scalar potential
A scalar potential is a fundamental concept in vector analysis and physics . The scalar potential is an example of a scalar field...
. A closed vector field (thought of as a 1-form) is one whose derivative (curl) vanishes, and is called an irrotational vector field
Irrotational vector field
In vector calculus a conservative vector field is a vector field which is the gradient of a function, known in this context as a scalar potential. Conservative vector fields have the property that the line integral from one point to another is independent of the choice of path connecting the two...
.
Thinking of a vector field as a 2-form instead, a closed vector field is one whose derivative (divergence
Divergence
In vector calculus, divergence is a vector operator that measures the magnitude of a vector field's source or sink at a given point, in terms of a signed scalar. More technically, the divergence represents the volume density of the outward flux of a vector field from an infinitesimal volume around...
) vanishes, and is called an incompressible flow
Incompressible flow
In fluid mechanics or more generally continuum mechanics, incompressible flow refers to flow in which the material density is constant within an infinitesimal volume that moves with the velocity of the fluid...
(sometimes solenoidal vector field).
Conservative and incompressible vector fields generalize to n-dimensions (gradient and divergence generalize to n dimensions); curl and hence irrotational does not generalize in this way.
Poincaré lemma
The Poincaré lemma states that if X is a contractibleContractible space
In mathematics, a topological space X is contractible if the identity map on X is null-homotopic, i.e. if it is homotopic to some constant map. Intuitively, a contractible space is one that can be continuously shrunk to a point....
open subset of Rn, any smooth closed p-form α defined on X is exact, for any integer p > 0 (this has content only when p ≤ n).
Contractibility means that there is a homotopy
Homotopy
In topology, two continuous functions from one topological space to another are called homotopic if one can be "continuously deformed" into the other, such a deformation being called a homotopy between the two functions...
Ft : X×[0,1] → X that continuously deforms X to a point. Thus every cycle c in X is the boundary of some "cone"; one may take the cone to be the image of c under the homotopy. A dual version of this gives the Poincaré lemma.
More specifically, we associate to X the cylinder X×[0,1]. Identify the top and bottom of the cylinder with the maps j1(x) = (x, 1) and j0(x) = (x, 0) respectively. On the differential forms, the induced maps j1* and j0* are related by a cochain homotopy K:

Let Ωp(X) denote the p-forms on X. The map K: Ωp + 1( X×[0,1] ) → Ωp(X) is the dual of the cylinder map and defined by

where dxp is a monomial p-form with no dt in it. So if F is a homotopy deforming X to a point Q, then

On forms,

Inserting these two equations into the cochain homotopy equation proves the Poincaré lemma.
Formulation as cohomology
When the difference of two closed forms is an exact form, they are said to be cohomologous to each other. That is, if ζ and η are closed forms, and one can find some β such that
then one says that ζ and η are cohomologous to each other. Exact forms are sometimes said to be cohomologous to zero. The set of all forms cohomologous to a given form (and thus to each other) is called a de Rham cohomology
De Rham cohomology
In mathematics, de Rham cohomology is a tool belonging both to algebraic topology and to differential topology, capable of expressing basic topological information about smooth manifolds in a form particularly adapted to computation and the concrete representation of cohomology classes...
class; the general study of such classes is known as cohomology
Cohomology
In mathematics, specifically in algebraic topology, cohomology is a general term for a sequence of abelian groups defined from a co-chain complex. That is, cohomology is defined as the abstract study of cochains, cocycles, and coboundaries...
. It makes no real sense to ask whether a 0-form (smooth function) is exact, since d increases degree by 1; but the clues from topology suggest that only the zero function should be called "exact". The cohomology classes are identified with locally constant functions.
A corollary of the Poincaré lemma is that de Rham cohomology is homotopy-invariant. Non-contractible spaces need not have trivial de Rham cohomology. For instance, on the circle S1, parametrized by t in [0, 1], the closed 1-form dt is not exact.
Application in electrodynamics
In electrodynamics, the case of the magnetic field produced by a stationary electrical current is important. There one deals with the vector potential
produced by a stationary electrical current is important. There one deals with the vector potentialVector potential
In vector calculus, a vector potential is a vector field whose curl is a given vector field. This is analogous to a scalar potential, which is a scalar field whose negative gradient is a given vector field....
 of this field. This case corresponds to k=2, and the defining region is the full
of this field. This case corresponds to k=2, and the defining region is the full  The current-density vector is
The current-density vector is  It corresponds to the current two-form
It corresponds to the current two-form
For the magnetic field
 one has analogous results: it corresponds to the induction two-form
one has analogous results: it corresponds to the induction two-form  and can be derived from the vector potential
and can be derived from the vector potential  , or the corresponding one-form
, or the corresponding one-form  ,
,
Thereby the vector potential
 corresponds to the potential one-form
corresponds to the potential one-form
The closedness of the magnetic-induction two-form corresponds to the property of the magnetic field that it is source-free:
 i.e. there are no magnetic monopole
i.e. there are no magnetic monopoleMonopole
Monopole may refer to:*Magnetic monopole, or Dirac monopole, a hypothetical particle that may be loosely described as a magnet with only one pole, or related concepts in physics and mathematics:...
s.
In a special gauge,
 , this implies for i = 1, 2, 3
, this implies for i = 1, 2, 3
(Here
 is a constant, the magnetic vacuum permeability.)
is a constant, the magnetic vacuum permeability.)This equation is remarkable, because it corresponds completely to a well-known formula for the electrical field
 , namely for the electrostatic Coulomb potential
, namely for the electrostatic Coulomb potential  of a charge density
of a charge density  . At this place one can already guess that
. At this place one can already guess that and
and 
 and
and 
 and
and 
can be unified to quantities with six rsp. four nontrivial components, which is the basis of the relativistic invariance of the Maxwell equations.
If the condition of stationarity is left, on the l.h.s. of the above-mentioned equation one must add, in the equations for
 to the three space coordinates, as a fourth variable also the time t, whereas on the r.h.s., in
to the three space coordinates, as a fourth variable also the time t, whereas on the r.h.s., in  the so-called "retarded time",
the so-called "retarded time",  must be used, i.e. it is added to the argument of the current-density. Finally, as before, one integrates over the three primed space coordinates. (As usual
must be used, i.e. it is added to the argument of the current-density. Finally, as before, one integrates over the three primed space coordinates. (As usualc is the vacuum velocity of light.)

