
Dicyclic group
Encyclopedia
In group theory
, a dicyclic group (notation Dicn) is a member of a class of non-abelian group
s of order 4n (n > 1). It is an extension
of the cyclic group of order 2 by a cyclic group of order 2n, giving the name di-cyclic. In the notation of exact sequence
s of groups, this extension can be expressed as:

More generally, given any finite abelian group with an order-2 element, one can define a dicyclic group.
of the unit quaternion
s generated by

More abstractly, one can define the dicyclic group Dicn as any group having the presentation

Some things to note which follow from this definition:
Thus, every element of Dicn can be uniquely written as akxj, where 0 ≤ k < 2n and j = 0 or 1. The multiplication rules are given by
It follows that Dicn has order
4n.
When n = 2, the dicyclic group is isomorphic to the quaternion group
Q. More generally, when n is a power of 2, the dicyclic group is isomorphic to the generalized quaternion group.
Let A = <a> be the subgroup of Dicn generated
by a. Then A is a cyclic group of order 2n, so [Dicn:A] = 2. As a subgroup of index
2 it is automatically a normal subgroup
. The quotient group Dicn/A is a cyclic group of order 2.
Dicn is solvable
; note that A is normal, and being abelian, is itself solvable.
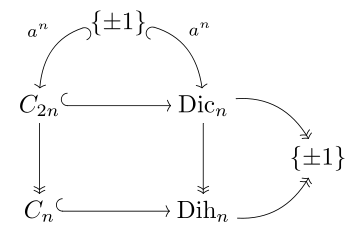
The dicyclic group is a binary polyhedral group — it is one of the classes of subgroups of the Pin group
Pin−(2), which is a subgroup of the Spin group Spin(3) — and in this context is known as the binary dihedral group.
The connection with the binary cyclic group C2n, the cyclic group Cn, and the dihedral group
Dihn of order 2n is illustrated in the diagram at right, and parallels the corresponding diagram for the Pin group.
There is a superficial resemblance between the dicyclic groups and dihedral group
s; both are a sort of "mirroring" of an underlying cyclic group. But the presentation of a dihedral group would have x2 = 1, instead of x2 = an; and this yields a different structure. In particular, Dicn is not a semidirect product
of A and <x>, since A ∩ <x> is not trivial.
The dicyclic group has a unique involution (i.e. an element of order 2), namely x2 = an. Note that this element lies in the center of Dicn. Indeed, the center consists solely of the identity element and x2. If we add the relation x2 = 1 to the presentation of Dicn one obtains a presentation of the dihedral group
Dih2n, so the quotient group Dicn/<x2> is isomorphic to Dihn.
There is a natural 2-to-1 homomorphism
from the group of unit quaternions to the 3-dimensional rotation group
described at quaternions and spatial rotation
s. Since the dicyclic group can be embedded inside the unit quaternions one can ask what the image of it is under this homomorphism. The answer is the just the dihedral symmetry group Dihn. For this reason the dicyclic group is also known as the binary dihedral group. Note that the dicyclic group does not contain any subgroup isomorphic to Dihn.
The analogous pre-image construction, using Pin+(2) instead of Pin−(2), yields another dihedral group, Dih2n, rather than a dicyclic group.
, having a specific element y in A with order 2. A group G is called a generalized dicyclic group, written as Dic(A, y), if it is generated by A and an additional element x, and in addition we have that [G:A] = 2, x2 = y, and for all a in A, x−1ax = a−1.
Since for a cyclic group of even order, there is always a unique element of order 2, we can see that dicyclic groups are just a specific type of generalized dicyclic group.
Group theory
In mathematics and abstract algebra, group theory studies the algebraic structures known as groups.The concept of a group is central to abstract algebra: other well-known algebraic structures, such as rings, fields, and vector spaces can all be seen as groups endowed with additional operations and...
, a dicyclic group (notation Dicn) is a member of a class of non-abelian group
Group (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
s of order 4n (n > 1). It is an extension
Group extension
In mathematics, a group extension is a general means of describing a group in terms of a particular normal subgroup and quotient group. If Q and N are two groups, then G is an extension of Q by N if there is a short exact sequence...
of the cyclic group of order 2 by a cyclic group of order 2n, giving the name di-cyclic. In the notation of exact sequence
Exact sequence
An exact sequence is a concept in mathematics, especially in homological algebra and other applications of abelian category theory, as well as in differential geometry and group theory...
s of groups, this extension can be expressed as:

More generally, given any finite abelian group with an order-2 element, one can define a dicyclic group.
Definition
For each integer n > 1, the dicyclic group Dicn can be defined as the subgroupSubgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
of the unit quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s generated by

More abstractly, one can define the dicyclic group Dicn as any group having the presentation
Presentation of a group
In mathematics, one method of defining a group is by a presentation. One specifies a set S of generators so that every element of the group can be written as a product of powers of some of these generators, and a set R of relations among those generators...

Some things to note which follow from this definition:
- x4 = 1
- x2ak = ak+n = akx2
- if j = ±1, then xjak = a-kxj.
- akx−1 = ak−nanx−1 = ak−nx2x−1 = ak−nx.
Thus, every element of Dicn can be uniquely written as akxj, where 0 ≤ k < 2n and j = 0 or 1. The multiplication rules are given by
It follows that Dicn has order
Order (group theory)
In group theory, a branch of mathematics, the term order is used in two closely related senses:* The order of a group is its cardinality, i.e., the number of its elements....
4n.
When n = 2, the dicyclic group is isomorphic to the quaternion group
Quaternion group
In group theory, the quaternion group is a non-abelian group of order eight, isomorphic to a certain eight-element subset of the quaternions under multiplication...
Q. More generally, when n is a power of 2, the dicyclic group is isomorphic to the generalized quaternion group.
Properties
For each n > 1, the dicyclic group Dicn is a non-abelian group of order 4n. ("Dic1" is C4, the cyclic group of order 4, which is abelian, and is not considered dicyclic.)Let A = <a> be the subgroup of Dicn generated
Generating set of a group
In abstract algebra, a generating set of a group is a subset that is not contained in any proper subgroup of the group. Equivalently, a generating set of a group is a subset such that every element of the group can be expressed as the combination of finitely many elements of the subset and their...
by a. Then A is a cyclic group of order 2n, so [Dicn:A] = 2. As a subgroup of index
Index of a subgroup
In mathematics, specifically group theory, the index of a subgroup H in a group G is the "relative size" of H in G: equivalently, the number of "copies" of H that fill up G. For example, if H has index 2 in G, then intuitively "half" of the elements of G lie in H...
2 it is automatically a normal subgroup
Normal subgroup
In abstract algebra, a normal subgroup is a subgroup which is invariant under conjugation by members of the group. Normal subgroups can be used to construct quotient groups from a given group....
. The quotient group Dicn/A is a cyclic group of order 2.
Dicn is solvable
Solvable group
In mathematics, more specifically in the field of group theory, a solvable group is a group that can be constructed from abelian groups using extensions...
; note that A is normal, and being abelian, is itself solvable.
Binary dihedral group

The dicyclic group is a binary polyhedral group — it is one of the classes of subgroups of the Pin group
Pin group
In mathematics, the pin group is a certain subgroup of the Clifford algebra associated to a quadratic space. It maps 2-to-1 to the orthogonal group, just as the spin group maps 2-to-1 to the special orthogonal group....
Pin−(2), which is a subgroup of the Spin group Spin(3) — and in this context is known as the binary dihedral group.
The connection with the binary cyclic group C2n, the cyclic group Cn, and the dihedral group
Dihedral group
In mathematics, a dihedral group is the group of symmetries of a regular polygon, including both rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry.See also: Dihedral symmetry in three...
Dihn of order 2n is illustrated in the diagram at right, and parallels the corresponding diagram for the Pin group.
There is a superficial resemblance between the dicyclic groups and dihedral group
Dihedral group
In mathematics, a dihedral group is the group of symmetries of a regular polygon, including both rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry.See also: Dihedral symmetry in three...
s; both are a sort of "mirroring" of an underlying cyclic group. But the presentation of a dihedral group would have x2 = 1, instead of x2 = an; and this yields a different structure. In particular, Dicn is not a semidirect product
Semidirect product
In mathematics, specifically in the area of abstract algebra known as group theory, a semidirect product is a particular way in which a group can be put together from two subgroups, one of which is a normal subgroup. A semidirect product is a generalization of a direct product...
of A and <x>, since A ∩ <x> is not trivial.
The dicyclic group has a unique involution (i.e. an element of order 2), namely x2 = an. Note that this element lies in the center of Dicn. Indeed, the center consists solely of the identity element and x2. If we add the relation x2 = 1 to the presentation of Dicn one obtains a presentation of the dihedral group
Dihedral group
In mathematics, a dihedral group is the group of symmetries of a regular polygon, including both rotations and reflections. Dihedral groups are among the simplest examples of finite groups, and they play an important role in group theory, geometry, and chemistry.See also: Dihedral symmetry in three...
Dih2n, so the quotient group Dicn/<x2> is isomorphic to Dihn.
There is a natural 2-to-1 homomorphism
Homomorphism
In abstract algebra, a homomorphism is a structure-preserving map between two algebraic structures . The word homomorphism comes from the Greek language: ὁμός meaning "same" and μορφή meaning "shape".- Definition :The definition of homomorphism depends on the type of algebraic structure under...
from the group of unit quaternions to the 3-dimensional rotation group
Rotation group
In mechanics and geometry, the rotation group is the group of all rotations about the origin of three-dimensional Euclidean space R3 under the operation of composition. By definition, a rotation about the origin is a linear transformation that preserves length of vectors and preserves orientation ...
described at quaternions and spatial rotation
Quaternions and spatial rotation
Unit quaternions provide a convenient mathematical notation for representing orientations and rotations of objects in three dimensions. Compared to Euler angles they are simpler to compose and avoid the problem of gimbal lock. Compared to rotation matrices they are more numerically stable and may...
s. Since the dicyclic group can be embedded inside the unit quaternions one can ask what the image of it is under this homomorphism. The answer is the just the dihedral symmetry group Dihn. For this reason the dicyclic group is also known as the binary dihedral group. Note that the dicyclic group does not contain any subgroup isomorphic to Dihn.
The analogous pre-image construction, using Pin+(2) instead of Pin−(2), yields another dihedral group, Dih2n, rather than a dicyclic group.
Generalizations
Let A be an abelian groupAbelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
, having a specific element y in A with order 2. A group G is called a generalized dicyclic group, written as Dic(A, y), if it is generated by A and an additional element x, and in addition we have that [G:A] = 2, x2 = y, and for all a in A, x−1ax = a−1.
Since for a cyclic group of even order, there is always a unique element of order 2, we can see that dicyclic groups are just a specific type of generalized dicyclic group.
See also
- binary polyhedral group
- binary cyclic group
- binary tetrahedral group
- binary octahedral group
- binary icosahedral group





