Cardioid
Encyclopedia
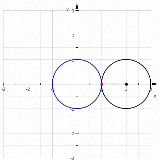
A cardioid is a plane curve
traced by a point on the perimeter of a circle that is rolling around a fixed circle of the same radius. It is therefore a type of limaçon
and can also be defined as an epicycloid
having a single cusp
. It is also a type of sinusoidal spiral
, and an inverse curve
of the parabola
with the focus as the center of inversion.
The name was coined by de Castillon
in 1741 but had been the subject of study decades beforehand. Named for its heart-like form, it is shaped more like the outline of the cross section of a round apple
without the stalk.
A cardioid microphone exhibits an acoustic
pickup pattern that, when graphed in two dimensions, resembles a cardioid, (any 2d plane containing the 3d straight line of the microphone body.) In three dimensions, the cardioid is shaped like an apple centred around the microphone which is the "stalk" of the apple.
s:

In the complex plane
this becomes
Here a is the radius of the circles which generate the curve, and the fixed circle is centered at the origin. The point generating the curve touches the fixed circle at (a, 0), the cusp. The parameter t can be eliminated giving
or, in rectangular coordinates,
These equations can be simplified somewhat by shifting the fixed circle to the right a units and choosing the point on the rolling circle chosen so it touches the fixed circle at the origin; this changes the orientation of the curve so that the cusp is on the left. The parametric equations are then:

or, in the complex plane,
With the substitution u=tan t/2,
giving a rational parameterization:
or

The parametrization can also be written
and in this form it is apparent that the equation for this cardioid may be written in polar coordinates as
where θ replaces the parameter t.
This can also be written
which implies that the curve is a member of the family of sinusoidal spiral
s.
In Cartesian coordinates, the equation for this cardioid is

or 6 times the area of the circles used in the construction.
The arc length
of a cardioid can be computed exactly, a rarity for algebraic curves. The total length is .
.
for a parabola
. Specifically, if a parabola is inverted across any circle
whose center lies at the focus of the parabola, the result is a cardioid. The cusp of the resulting cardioid will lie at the center of the circle, and corresponds to the vanishing point
of the parabola.
In terms of stereographic projection
, this says that a parabola in the Euclidean plane is the projection of a cardioid drawn on the sphere
whose cusp is at the north pole.
Not every inverse curve of a parabola is a cardioid. For example, if a parabola is inverted across a circle whose center lies at the vertex of the parabola, then the result is a cissoid of Diocles
.
The picture to the right shows the parabola with polar equation
In Cartesian coordinates, this is the parabola . When this parabola is inverted across the unit circle
. When this parabola is inverted across the unit circle
, the result is a cardioid with the reciprocal
equation
, the image
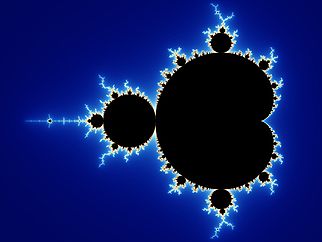
of any circle through the origin under the map is a cardioid. One application of this result is that the boundary of the central bulb of the Mandelbrot set
is a cardioid. One application of this result is that the boundary of the central bulb of the Mandelbrot set
is a cardioid given by the equation

The Mandelbrot set contains an infinite number of slightly distorted copies of itself and the central bulb of any of these smaller copies is an approximate cardioid.
can take the shape of cardioids. The catacaustic of a circle with respect to a point on the circumference is a cardioid. Also, the catacaustic of a cone with respect to rays parallel to a generating line is a surface whose cross section is a cardioid. This can be seen, as in the photograph to the right, in a conical cup partially filled with liquid when a light is shining from a distance and at an angle equal to the angle of the cone. The shape of the curve at the bottom of a cylindrical cup is a nephroid
, which looks quite similar.
Plane curve
In mathematics, a plane curve is a curve in a Euclidean plane . The most frequently studied cases are smooth plane curves , and algebraic plane curves....
traced by a point on the perimeter of a circle that is rolling around a fixed circle of the same radius. It is therefore a type of limaçon
Limaçon
In geometry, a limaçon or limacon , also known as a limaçon of Pascal, is defined as a roulette formed when a circle rolls around the outside of a circle of equal radius. It can also be defined as the roulette formed when a circle rolls around a circle with half its radius so that the smaller...
and can also be defined as an epicycloid
Epicycloid
In geometry, an epicycloid is a plane curve produced by tracing the path of a chosen point of a circle — called an epicycle — which rolls without slipping around a fixed circle...
having a single cusp
Cusp (singularity)
In the mathematical theory of singularities a cusp is a type of singular point of a curve. Cusps are local singularities in that they are not formed by self intersection points of the curve....
. It is also a type of sinusoidal spiral
Sinusoidal spiral
In geometry, the sinusoidal spirals are a family of curves defined by the equation in polar coordinatesr^n = a^n \cos\,where a is a nonzero constant and n is a rational number other than 0...
, and an inverse curve
Inverse curve
In geometry, an inverse curve of a given curve C is the result of applying an inverse operation to C. Specifically, with respect to a fixed circle with center O and radius k the inverse of a point Q is the point P for which P lies on the ray OQ and OP·PQ = k2...
of the parabola
Parabola
In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...
with the focus as the center of inversion.
The name was coined by de Castillon
Giovanni Salvemini
Giovanni Francesco Mauro Melchiorre Salvemini di Castiglione FRS was an Italian mathematician and astronomer.-Life:...
in 1741 but had been the subject of study decades beforehand. Named for its heart-like form, it is shaped more like the outline of the cross section of a round apple
Apple
The apple is the pomaceous fruit of the apple tree, species Malus domestica in the rose family . It is one of the most widely cultivated tree fruits, and the most widely known of the many members of genus Malus that are used by humans. Apple grow on small, deciduous trees that blossom in the spring...
without the stalk.
A cardioid microphone exhibits an acoustic
Acoustics
Acoustics is the interdisciplinary science that deals with the study of all mechanical waves in gases, liquids, and solids including vibration, sound, ultrasound and infrasound. A scientist who works in the field of acoustics is an acoustician while someone working in the field of acoustics...
pickup pattern that, when graphed in two dimensions, resembles a cardioid, (any 2d plane containing the 3d straight line of the microphone body.) In three dimensions, the cardioid is shaped like an apple centred around the microphone which is the "stalk" of the apple.
Equations
Based on the rolling circle description, with the fixed circle having the origin as its center, and both circle having radius a, the cardioid is given by the following parametric equationParametric equation
In mathematics, parametric equation is a method of defining a relation using parameters. A simple kinematic example is when one uses a time parameter to determine the position, velocity, and other information about a body in motion....
s:


In the complex plane
Complex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
this becomes

Here a is the radius of the circles which generate the curve, and the fixed circle is centered at the origin. The point generating the curve touches the fixed circle at (a, 0), the cusp. The parameter t can be eliminated giving

or, in rectangular coordinates,

These equations can be simplified somewhat by shifting the fixed circle to the right a units and choosing the point on the rolling circle chosen so it touches the fixed circle at the origin; this changes the orientation of the curve so that the cusp is on the left. The parametric equations are then:


or, in the complex plane,

With the substitution u=tan t/2,

giving a rational parameterization:

or


The parametrization can also be written

and in this form it is apparent that the equation for this cardioid may be written in polar coordinates as

where θ replaces the parameter t.
This can also be written

which implies that the curve is a member of the family of sinusoidal spiral
Sinusoidal spiral
In geometry, the sinusoidal spirals are a family of curves defined by the equation in polar coordinatesr^n = a^n \cos\,where a is a nonzero constant and n is a rational number other than 0...
s.
In Cartesian coordinates, the equation for this cardioid is

Metrical properties
The area enclosed by a cardioid can be computed from the polar equation:
or 6 times the area of the circles used in the construction.
The arc length
Arc length
Determining the length of an irregular arc segment is also called rectification of a curve. Historically, many methods were used for specific curves...
of a cardioid can be computed exactly, a rarity for algebraic curves. The total length is
 .
.Inverse curve
The cardioid is one possible inverse curveInverse curve
In geometry, an inverse curve of a given curve C is the result of applying an inverse operation to C. Specifically, with respect to a fixed circle with center O and radius k the inverse of a point Q is the point P for which P lies on the ray OQ and OP·PQ = k2...
for a parabola
Parabola
In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...
. Specifically, if a parabola is inverted across any circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
whose center lies at the focus of the parabola, the result is a cardioid. The cusp of the resulting cardioid will lie at the center of the circle, and corresponds to the vanishing point
Vanishing point
A vanishing point is a point in a perspective drawing to which parallel lines not parallel to the image plane appear to converge. The number and placement of the vanishing points determines which perspective technique is being used...
of the parabola.
In terms of stereographic projection
Stereographic projection
The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it...
, this says that a parabola in the Euclidean plane is the projection of a cardioid drawn on the sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
whose cusp is at the north pole.
Not every inverse curve of a parabola is a cardioid. For example, if a parabola is inverted across a circle whose center lies at the vertex of the parabola, then the result is a cissoid of Diocles
Cissoid of Diocles
In geometry, the cissoid of Diocles is a cubic plane curve notable for the property that it can be used to construct two mean proportionals to a given ratio. In particular, it can be used to double a cube. It can be defined as the cissoid of a circle and a line tangent to it with respect to the...
.
The picture to the right shows the parabola with polar equation

In Cartesian coordinates, this is the parabola
 . When this parabola is inverted across the unit circle
. When this parabola is inverted across the unit circleUnit circle
In mathematics, a unit circle is a circle with a radius of one. Frequently, especially in trigonometry, "the" unit circle is the circle of radius one centered at the origin in the Cartesian coordinate system in the Euclidean plane...
, the result is a cardioid with the reciprocal
Multiplicative inverse
In mathematics, a multiplicative inverse or reciprocal for a number x, denoted by 1/x or x−1, is a number which when multiplied by x yields the multiplicative identity, 1. The multiplicative inverse of a fraction a/b is b/a. For the multiplicative inverse of a real number, divide 1 by the...
equation


Cardioids in complex analysis
In complex analysisComplex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is useful in many branches of mathematics, including number theory and applied mathematics; as well as in physics,...
, the image
Image (mathematics)
In mathematics, an image is the subset of a function's codomain which is the output of the function on a subset of its domain. Precisely, evaluating the function at each element of a subset X of the domain produces a set called the image of X under or through the function...
of any circle through the origin under the map
 is a cardioid. One application of this result is that the boundary of the central bulb of the Mandelbrot set
is a cardioid. One application of this result is that the boundary of the central bulb of the Mandelbrot setMandelbrot set
The Mandelbrot set is a particular mathematical set of points, whose boundary generates a distinctive and easily recognisable two-dimensional fractal shape...
is a cardioid given by the equation
Parametric equation
In mathematics, parametric equation is a method of defining a relation using parameters. A simple kinematic example is when one uses a time parameter to determine the position, velocity, and other information about a body in motion....

The Mandelbrot set contains an infinite number of slightly distorted copies of itself and the central bulb of any of these smaller copies is an approximate cardioid.
Caustics
Certain causticsCaustic (mathematics)
In differential geometry and geometric optics, a caustic is the envelope of rays either reflected or refracted by a manifold. It is related to the optical concept of caustics...
can take the shape of cardioids. The catacaustic of a circle with respect to a point on the circumference is a cardioid. Also, the catacaustic of a cone with respect to rays parallel to a generating line is a surface whose cross section is a cardioid. This can be seen, as in the photograph to the right, in a conical cup partially filled with liquid when a light is shining from a distance and at an angle equal to the angle of the cone. The shape of the curve at the bottom of a cylindrical cup is a nephroid
Nephroid
The nephroid is a plane curve whose name means kidney-shaped Although the term nephroid was used to describe other curves, it was applied to the curve in this article by Proctor in 1878. This and the information below may be verified in Lockwood, pp...
, which looks quite similar.
See also
- NephroidNephroidThe nephroid is a plane curve whose name means kidney-shaped Although the term nephroid was used to describe other curves, it was applied to the curve in this article by Proctor in 1878. This and the information below may be verified in Lockwood, pp...
- DeltoidDeltoid curveIn geometry, a deltoid, also known as a tricuspoid or Steiner curve, is a hypocycloid of three cusps. In other words, it is the roulette created by a point on the circumference of a circle as it rolls without slipping along the inside of a circle with three times its radius...
- Wittgenstein's rodWittgenstein's rodWittgenstein's rod is a geometry problem attributed to 20th century philosopher Ludwig Wittgenstein.The set-up is as follows: a rod passes through a sleeve, in which it can smoothly slide. The sleeve is tangentially affixed to a wall at its lengthwise center, forming a pivot. One end of the rod is...
- Cardioid microphone
- LemniscateLemniscateIn algebraic geometry, a lemniscate refers to any of several figure-eight or ∞ shaped curves. It may refer to:*The lemniscate of Bernoulli, often simply called the lemniscate, the locus of points whose product of distances from two foci equals the square of half the interfocal distance*The...
- Loop antennaLoop antennaA loop antenna is a radio antenna consisting of a loop of wire, tubing, or other electrical conductor with its ends connected to a balanced transmission line...
- Radio direction finderRadio direction finderA radio direction finder is a device for finding the direction to a radio source. Due to low frequency propagation characteristic to travel very long distances and "over the horizon", it makes a particularly good navigation system for ships, small boats, and aircraft that might be some distance...
- Radio direction finding
- Yagi antennaYagi antennaA Yagi-Uda array, commonly known simply as a Yagi antenna, is a directional antenna consisting of a driven element and additional parasitic elements...
External links
- Hearty Munching on Cardioids at cut-the-knotCut-the-knotCut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...
- Xah Lee, Cardioid (1998) (This site provides a number of alternative constructions).
- Jan Wassenaar, Cardioid, (2005)

