
Simple Lie group
Encyclopedia
In group theory
, a simple Lie group is a connected
non-abelian
Lie group
G which does not have nontrivial connected normal subgroups.
A simple Lie algebra is a non-abelian Lie algebra
whose only ideals are 0 and itself. A direct sum of simple Lie algebras is called a semisimple Lie algebra.
An equivalent definition of a simple Lie group follows from the Lie correspondence: a connected Lie group is simple if its Lie algebra
is simple. An important technical point is that
a simple Lie group may contain discrete normal subgroups, hence being a simple Lie group is different from being simple as an abstract group
.
Simple Lie groups include many classical Lie groups, which provide a group-theoretic underpinning for spherical geometry
, projective geometry
and related geometries in the sense of Felix Klein
's Erlangen programme. It emerged in the course of classification of simple Lie groups that there exist also several exceptional
possibilities not corresponding to any familiar geometry. These exceptional groups account for many special examples and configurations in other branches of mathematics, as well as contemporary theoretical physics
.
While the notion of a simple Lie group is satisfying from the axiomatic perspective, in applications of Lie theory, such as the theory of Riemannian symmetric space
s, somewhat more general notions of semisimple and reductive
Lie groups proved to be even more useful. In particular, every connected compact Lie group is reductive, and the study of representations of general reductive groups is a major branch of representation theory
.
The most common definition is the one above: simple Lie groups have to be connected, they are allowed to have non-trivial centers (possibly infinite), they need not be representable by finite matrices, and they must be non-abelian.
s. It is shown that a simple Lie group has a simple Lie algebra that will occur on the list given there, once it is complexified (that is, made into a complex vector space rather than a real one). This reduces the classification to two further matters.
, by computing the fundamental group
of G (an abelian group
: a Lie group is an H-space
). This was done by Élie Cartan
.
For an example, take the special orthogonal groups in even dimension. With the non-identity matrix −I in the center
, these aren't actually simple groups; and having a twofold spin cover, they aren't simply-connected either. They lie 'between' G* and G, in the notation above.
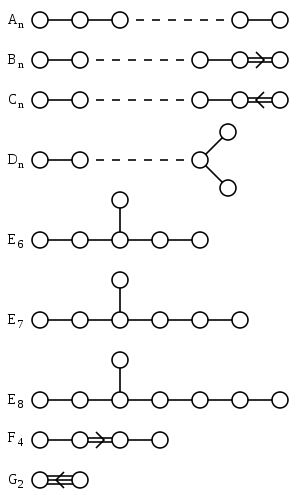
Ar corresponds to the special linear group
, SL(r+1)
.
Br corresponds to the special orthogonal group, SO(2r+1).
Cr corresponds to the symplectic group
, Sp(2r)
.
Dr corresponds to the special orthogonal group, SO(2r). Note that SO(4) is not a simple group, though. The Dynkin diagram has two nodes that are not connected. There is a surjective homomorphism from SO(3)* × SO(3)* to SO(4) given by quaternion
multiplication; see quaternions and spatial rotation. Therefore the simple groups here start with D3, which as a diagram straightens out to A3. With D4 there is an 'exotic' symmetry of the diagram, corresponding to so-called triality
.
. These cases are deemed 'exceptional
' because they do not fall into infinite series of groups of increasing dimension. From the point of view of each group taken separately, there is nothing so unusual about them. These exceptional groups were discovered around 1890 in the classification of the simple Lie algebras, over the complex numbers (Wilhelm Killing
, re-done by Élie Cartan
). For some time it was a research issue to find concrete ways in which they arise, for example as a symmetry group of a differential system.
See also E7½
whose Dynkin diagram only contain simple links, and therefore all the nonzero roots of the corresponding Lie algebra have the same length. The A, D and E series groups are all simply laced, but no group of type B, C, F, or G is simply laced.
Group theory
In mathematics and abstract algebra, group theory studies the algebraic structures known as groups.The concept of a group is central to abstract algebra: other well-known algebraic structures, such as rings, fields, and vector spaces can all be seen as groups endowed with additional operations and...
, a simple Lie group is a connected
Connected space
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint nonempty open subsets. Connectedness is one of the principal topological properties that is used to distinguish topological spaces...
non-abelian
Nonabelian group
In mathematics, a non-abelian group, also sometimes called a non-commutative group, is a group in which there are at least two elements a and b of G such that a * b ≠ b * a...
Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
G which does not have nontrivial connected normal subgroups.
A simple Lie algebra is a non-abelian Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
whose only ideals are 0 and itself. A direct sum of simple Lie algebras is called a semisimple Lie algebra.
An equivalent definition of a simple Lie group follows from the Lie correspondence: a connected Lie group is simple if its Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
is simple. An important technical point is that
a simple Lie group may contain discrete normal subgroups, hence being a simple Lie group is different from being simple as an abstract group
Simple group
In mathematics, a simple group is a nontrivial group whose only normal subgroups are the trivial group and the group itself. A group that is not simple can be broken into two smaller groups, a normal subgroup and the quotient group, and the process can be repeated...
.
Simple Lie groups include many classical Lie groups, which provide a group-theoretic underpinning for spherical geometry
Spherical geometry
Spherical geometry is the geometry of the two-dimensional surface of a sphere. It is an example of a geometry which is not Euclidean. Two practical applications of the principles of spherical geometry are to navigation and astronomy....
, projective geometry
Projective geometry
In mathematics, projective geometry is the study of geometric properties that are invariant under projective transformations. This means that, compared to elementary geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts...
and related geometries in the sense of Felix Klein
Felix Klein
Christian Felix Klein was a German mathematician, known for his work in group theory, function theory, non-Euclidean geometry, and on the connections between geometry and group theory...
's Erlangen programme. It emerged in the course of classification of simple Lie groups that there exist also several exceptional
Exceptional object
Many branches of mathematics study objects of a given type and prove a classification theorem. A common theme is that the classification results in a number of series of objects as well as a finite number of exceptions that don't fit into any series. These are known as exceptional...
possibilities not corresponding to any familiar geometry. These exceptional groups account for many special examples and configurations in other branches of mathematics, as well as contemporary theoretical physics
Theoretical physics
Theoretical physics is a branch of physics which employs mathematical models and abstractions of physics to rationalize, explain and predict natural phenomena...
.
While the notion of a simple Lie group is satisfying from the axiomatic perspective, in applications of Lie theory, such as the theory of Riemannian symmetric space
Riemannian symmetric space
In differential geometry, representation theory and harmonic analysis, a symmetric space is a smooth manifold whose group of symmetries contains an inversion symmetry about every point. There are two ways to formulate the inversion symmetry, via Riemannian geometry or via Lie theory...
s, somewhat more general notions of semisimple and reductive
Reductive group
In mathematics, a reductive group is an algebraic group G over an algebraically closed field such that the unipotent radical of G is trivial . Any semisimple algebraic group is reductive, as is any algebraic torus and any general linear group...
Lie groups proved to be even more useful. In particular, every connected compact Lie group is reductive, and the study of representations of general reductive groups is a major branch of representation theory
Representation theory
Representation theory is a branch of mathematics that studies abstract algebraic structures by representing their elements as linear transformations of vector spaces, and studiesmodules over these abstract algebraic structures...
.
Comments on the definition
Unfortunately there is no single standard definition of a simple Lie group. The definition given above is sometimes varied in the following ways:- Connectedness: Usually simple Lie groups are connected by definition. This excludes discrete simple groups (these are zero-dimensional Lie groups that are simpleSimple groupIn mathematics, a simple group is a nontrivial group whose only normal subgroups are the trivial group and the group itself. A group that is not simple can be broken into two smaller groups, a normal subgroup and the quotient group, and the process can be repeated...
as abstract groups) as well as disconnected orthogonal groupOrthogonal groupIn mathematics, the orthogonal group of degree n over a field F is the group of n × n orthogonal matrices with entries from F, with the group operation of matrix multiplication...
s. - Center: Usually simple Lie groups are allowed to have a discrete center; for example, SL2(R) has a center of order 2, but is still counted as a simple Lie group. If the center is non-trivial (and not the whole group) then the simple Lie group is not simple as an abstract group. Some authors require that the center of a simple Lie group be finite (or trivial); the universal cover of SL2(R) is an example of a simple Lie group with infinite center.
- R: Usually the group R of real numbers under addition (and its quotient R/Z) are not counted as simple Lie groups, even though they are connected and have a Lie algebra with no proper non-zero ideals. Occasionally authors define simple Lie groups in such a way that R is simple, though this sometimes seems to be an accident caused by overlooking this case.
- Matrix groups: Some authors restrict themselves to Lie groups that can be represented as groups of finite matrices. The metaplectic groupMetaplectic groupIn mathematics, the metaplectic group Mp2n is a double cover of the symplectic group Sp2n. It can be defined over either real or p-adic numbers...
is an example of a simple Lie group that cannot be represented in this way. - Complex Lie algebras: The definition of a simple Lie algebra is not stable under the extension of scalars. The complexificationComplexificationIn mathematics, the complexification of a real vector space V is a vector space VC over the complex number field obtained by formally extending scalar multiplication to include multiplication by complex numbers. Any basis for V over the real numbers serves as a basis for VC over the complex...
of a complex simple Lie algebra, such as sln(C) is semisimple, but not simple.
The most common definition is the one above: simple Lie groups have to be connected, they are allowed to have non-trivial centers (possibly infinite), they need not be representable by finite matrices, and they must be non-abelian.
Method of classification
Such groups are classified using the prior classification of the complex simple Lie algebras: for which see the page on root systemRoot system
In mathematics, a root system is a configuration of vectors in a Euclidean space satisfying certain geometrical properties. The concept is fundamental in the theory of Lie groups and Lie algebras...
s. It is shown that a simple Lie group has a simple Lie algebra that will occur on the list given there, once it is complexified (that is, made into a complex vector space rather than a real one). This reduces the classification to two further matters.
Real forms
The groups SO(p,q,R) and SO(p+q,R), for example, give rise to different real Lie algebras, but having the same Dynkin diagram. In general there may be different real forms of the same complex Lie algebra.Relationship of simple Lie algebras to groups
Secondly the Lie algebra only determines uniquely the simply connected (universal) cover G* of the component containing the identity of a Lie group G. It may well happen that G* isn't actually a simple group, for example having a non-trivial center. We have therefore to worry about the global topologyAlgebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
, by computing the fundamental group
Fundamental group
In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
of G (an abelian group
Abelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
: a Lie group is an H-space
H-space
In mathematics, an H-space is a topological space X together with a continuous map μ : X × X → X with an identity element e so that μ = μ = x for all x in X...
). This was done by Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
.
For an example, take the special orthogonal groups in even dimension. With the non-identity matrix −I in the center
Center (group theory)
In abstract algebra, the center of a group G, denoted Z,The notation Z is from German Zentrum, meaning "center". is the set of elements that commute with every element of G. In set-builder notation,...
, these aren't actually simple groups; and having a twofold spin cover, they aren't simply-connected either. They lie 'between' G* and G, in the notation above.
Classification by Dynkin diagram
According to Dynkin's classification, we have as possibilities these only, where n is the number of nodes:
A series
A1, A2, ...Ar corresponds to the special linear group
Special linear group
In mathematics, the special linear group of degree n over a field F is the set of n×n matrices with determinant 1, with the group operations of ordinary matrix multiplication and matrix inversion....
, SL(r+1)
Special linear group
In mathematics, the special linear group of degree n over a field F is the set of n×n matrices with determinant 1, with the group operations of ordinary matrix multiplication and matrix inversion....
.
B series
B2, B3, ...Br corresponds to the special orthogonal group, SO(2r+1).
C series
C2, C3, ...Cr corresponds to the symplectic group
Symplectic group
In mathematics, the name symplectic group can refer to two different, but closely related, types of mathematical groups, denoted Sp and Sp. The latter is sometimes called the compact symplectic group to distinguish it from the former. Many authors prefer slightly different notations, usually...
, Sp(2r)
Symplectic group
In mathematics, the name symplectic group can refer to two different, but closely related, types of mathematical groups, denoted Sp and Sp. The latter is sometimes called the compact symplectic group to distinguish it from the former. Many authors prefer slightly different notations, usually...
.
D series
D4, D5, ...Dr corresponds to the special orthogonal group, SO(2r). Note that SO(4) is not a simple group, though. The Dynkin diagram has two nodes that are not connected. There is a surjective homomorphism from SO(3)* × SO(3)* to SO(4) given by quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
multiplication; see quaternions and spatial rotation. Therefore the simple groups here start with D3, which as a diagram straightens out to A3. With D4 there is an 'exotic' symmetry of the diagram, corresponding to so-called triality
Triality
In mathematics, triality is a relationship between three vector spaces, analogous to the duality relation between dual vector spaces. Most commonly, it describes those special features of the Dynkin diagram D4 and the associated Lie group Spin, the double cover of 8-dimensional rotation group SO,...
.
Exceptional cases
For the so-called exceptional cases see G2, F4, E6, E7, and E8E8 (mathematics)
In mathematics, E8 is any of several closely related exceptional simple Lie groups, linear algebraic groups or Lie algebras of dimension 248; the same notation is used for the corresponding root lattice, which has rank 8...
. These cases are deemed 'exceptional
Exceptional object
Many branches of mathematics study objects of a given type and prove a classification theorem. A common theme is that the classification results in a number of series of objects as well as a finite number of exceptions that don't fit into any series. These are known as exceptional...
' because they do not fall into infinite series of groups of increasing dimension. From the point of view of each group taken separately, there is nothing so unusual about them. These exceptional groups were discovered around 1890 in the classification of the simple Lie algebras, over the complex numbers (Wilhelm Killing
Wilhelm Killing
Wilhelm Karl Joseph Killing was a German mathematician who made important contributions to the theories of Lie algebras, Lie groups, and non-Euclidean geometry....
, re-done by Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
). For some time it was a research issue to find concrete ways in which they arise, for example as a symmetry group of a differential system.
See also E7½
E7½ (Lie algebra)
In mathematics, the Lie algebra E7½ is a subalgebra of E8 containing E7 defined by Landsberg and Manivel in orderto fill the "hole" in a dimension formula for the exceptional series En of simple Lie algebras. This hole was observed by Cvitanovic, Deligne, Cohen and de Man...
Simply laced groups
A simply laced group is a Lie groupLie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
whose Dynkin diagram only contain simple links, and therefore all the nonzero roots of the corresponding Lie algebra have the same length. The A, D and E series groups are all simply laced, but no group of type B, C, F, or G is simply laced.
See also
- Cartan matrixCartan matrixIn mathematics, the term Cartan matrix has three meanings. All of these are named after the French mathematician Élie Cartan. In fact, Cartan matrices in the context of Lie algebras were first investigated by Wilhelm Killing, whereas the Killing form is due to Cartan.- Lie algebras :A generalized...
- Coxeter matrix
- Weyl groupWeyl groupIn mathematics, in particular the theory of Lie algebras, the Weyl group of a root system Φ is a subgroup of the isometry group of the root system. Specifically, it is the subgroup which is generated by reflections through the hyperplanes orthogonal to the roots, and as such is a finite reflection...
- Coxeter groupCoxeter groupIn mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...
- Kac–Moody algebraKac–Moody algebraIn mathematics, a Kac–Moody algebra is a Lie algebra, usually infinite-dimensional, that can be defined by generators and relations through a generalized Cartan matrix...
- Catastrophe theoryCatastrophe theoryIn mathematics, catastrophe theory is a branch of bifurcation theory in the study of dynamical systems; it is also a particular special case of more general singularity theory in geometry....

